Easy Reasoning analogy methods for problems of S S C Exams

Ten most Important questions of Reasoning Analogy of missing numbers have been discussed with their Solutions in an easy manners. These questions are very very important for competitive exams like SSC CGL , SSC CHSL And RRB NTPC Etc Because such types of problems are frequently asked in these exams.

Problem #1 

Starting from 5 and moving clockwise  we have these numbers 5, 10,  ?, 50, 122. 

so 5 can be written  as 2²+1 starting from 1st prime  number i. e.  Square of 1st  prime number  and plus 1

10 can be written  as 3²+1 , Square  of 2nd prime number  and plus 1.

50 can be written as 7² +1, Square  of  4th prime number  and plus 1.

Similarly 122 can be written as 11² +1, Square  of  5th prime number  and plus 1.

Hence ? can be replaced as 5² +1, Square  of  3rd prime number ( 3 )  and plus 1.

So

1st Term = 5 = 2² + 1

2nd Term = 10 = 3² + 1

3rd Term = ? = 5² + 1

4th Term = 50 = 7² + 1

5th Term = 122 = 11² + 1

Therefore  Required  number  is 26.

Problem #2 

In this figure largest numbers are appearing in last row. so we should search the relation column wise .

In 1st column we have to search the relationship  between  9 and 6 to give 45. similarly in 2nd column we have to find relationship between 12 and 7 to give 95. And same logic we shall apply in 3rd columns. 

1st Column 

In 1st columns if we add and subtract both numbers  then multiply  it then we shall have 45 as follows

we have two numbers in 1st column  9 and 6 .

(9 + 6) × (9 - 6) = (15 ) × ( 3 ) = 45

2nd Column

And again in 2nd  columns if we add and subtract both numbers   of 2nd column and then multiply  these with each others  then we shall have 45 like this 

we have two numbers in 1st column  9 and 6 .

(12 + 7) × (12 - 7) = (19) × (5) = 95

3rd Column

Same logic will be applied for 3rd column ,we add and subtract both numbers   of 3rd column and then multiply  these with each others

( 8 + 3 ) × ( 8 -3 ) = (11 × 5 ) = 55

Hence 55 shall replace  "?" in the given figure 

Test your Reasoning ability

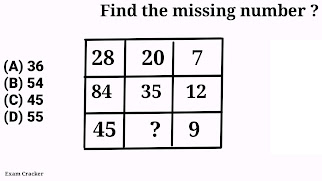
Problem #3

Since largest numbers  in all the three rows lie in 1st column of the given figure.

It means we have to search a relation between  20 and 7 to give 28 in 1st row. and similar relation must be between 35 and 12 to give 84 .

1st Row

So in 1st row if we multiply both numbers with each others and divide the result  with 5 we shall get 28 like this 20 × 7 =140 

Now divide 140 with 5 such that 140/5 =  28 

2nd Row

So in 2nd row if we have to multiply both numbers with each others and divide the result  with 5 we shall get 28 like this 35 × 12 = 420 .

3rd Row

Now divide 420 with 5 such that 420 ÷ 5 =  84

Same logic we have to apply in 3rd row to get answer 45 . So If we  multiply  9 with 45 we shall get 275 then we have to divide 405 with 5 to give  , It will replace ? question mark as follows 

9 × 25 = 225 ÷  5 = 45

 So  45 shall be the right number to replace question  mark .

Problem #4

Since largest numbers appears on 3rd columns therefore the solution must be row wise, if we treat a and b as 1st and 2nd numbers then 3rd number which is our desired numbers must be equal to (a-1)×(b) i .e. product/ multiplication  of (a-1)  and b. Hence 3rd element of 1st row must be 

(8 - 1) × (3) = 7 × 3 = 21

3rd element of 2nd row must be 

(6 - 1) × (5) = 5 × 5 = 25

So 3td element of 3rd row must be 

(12 - 1) × (2) = 11 × 2= 22

So 22 will replace "?" Question  mark.

Problem #5

In 1st column 5 × ( 6 +7 ) = 5 × 13 = 65

In 2nd column 4 × ( 3 +2 ) = 4 × 5 = 20

So same formula will be used in 3rd column

 In 3rd  column.  9 × ( ? +4 ) = 45

                                     ( ? +4 ) = 45/9 =5

                                              ? = 5 - 4  = 1

So required answer is   " 1 ".

Problem #6

Multiplying 1st three elements of all the columns to get 4th elements 

1 × 8 × 9 = 72

3 × 6 × 5 = 90 

2 × 7 × ? = 56

This implies ? = 4 

So ? will be replaced by 4

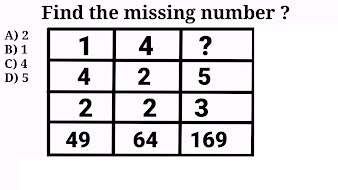
Problem #7

In 1st column square of three numbers i.e. 

( 1 + 4 + 2 )²  = 7²  = 49

In 2nd column square of three  numbers i.e. (4 + 2 +2)² = (8)² = 64

In 3rd column the square of  ( ? + 5 + 3 )²  must be 169

this implies  ( ? + 8)² = 169

( ? + 8 ) = 13

? = 5

Required answer is 5

Problem #8

If we calculate the sum of 1st column ,2nd column or  2nd row and 3rd row ,then it is found 25 in both the cases. So total of all rows and all the columns must be 25 . It can be seen that if we put "11"  in place of question mark then total of all the rows and columns is  25 . 

 Row Wise 

6 + 8 + ? = 9 + 3 + 13 = 10 + 14 +1 = 25 

Column Wise 

6 + 9 + 10 = 8 + 3 + 14 = ? + 13 +11 = 25 

? = 24  = 25

? = 25 - 24 

? = 1

So required answer is 1

Problem #9

Since biggest numbers are in the fourth columns of every row. So if we multiply 1st three numbers and then add 4th number to it ,we shall have 5th number in every row .

( 4 × 3 × 2 ) + 8 = 24 + 8 = 32

( 5 × 3 × 1 ) + 9 = 15 + 9 = 24

( 7 × 3 × 3 ) + 7 = 63 + 7 = 70

Similarly when we multiply first three numbers and then adding fourth number to it in the last row we shall have fourth number in the last row like this

( 2 × 9 × 4 ) + 12 = 72 + 12 = 84

So 84 will replace question mark " ?"

So required answer is 84

Problem #10

1st Row 

Multiply 3 and 2 then add one less than the 2nd number to it

( 3 × 2 ) + ( 2 - 1 ) = 6 + 1 = 1 

2nd Row 

Multiply 5 and 4 then add one less than the 2nd number to it

( 5 × 4  ) + ( 4 - 1 ) = 20 + 3 = 23

3rd Row

Multiply 7 and 6 then add one less than the 2nd number to it

( 7 × 6 ) + ( 6 - 1 ) = 42 + 5 = 47

4th Row

( 9 × 8 ) + ( 8 - 1) = 72 + 7 = 79

5th Row

( 10 × 9 ) + ( 9 - 1 ) = 90 + 8 = 98
So 98 will replace. " ? " 

How-to-Solve-hard-and-impossible-Puzzles

To Crack Any Competitive Exam Visit This You Tube Channel For Maths And Reasoning 

EXAM CRACKER

My previous Post

How to Solve linear equations of three VARIABLES BY MATRIX-METHOD

 How to find area bounded by two circles 

 How to integrate integral with square root in numerator     

How to find area of the circles which is interior to the parabola


How to find common area of two parabolas.

Final Words

  Thanks for watching and responding to this post of reasoning based on mathematics and number sequence puzzle solver.

Appeal 

If you are a mathematician Don't forget to visit my Mathematics You tube channel ,Mathematics Website and Mathematics Facebook Page , whose links are given below

Maths Website , Facebook Page   and     You Tube Channel

Also watch this video