Ten most important problems of Reasoning for competitive exam Part1
Ten most important problems of Reasoning for competitive exam which includes missing numbers and missing terms and their solutions in reasoning analogy . These questions are very very important for upcoming competitive exams like SSC CGL ,SSC CHSL and RRB NTPC Etc
5 : 100 :: 7 : ?
Problem # 3
6 : 18 :: 4 : ?
18 : 30 :: 36 : ?
Problem # 5
12 : 20 :: 30 : ?
Method 2
Problem # 6
12 : 54 :: 15 : ?
Problem # 7
6 : 5 :: 8 : ?
Problem # 8
29 : 319 :: 23 : ?
Problem # 9
6 : 64 :: 11 : ?
Problem # 10
36 : 50 :: 64 : ?
To buy cell phones and books for competitive Exams click here
Ten most important problems of Reasoning for competitive exams
Solution
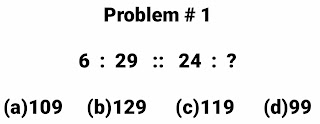
6 is related to 29 in the same way 24 will be related to ? , It means we have to apply same mathematical operations to 24 to get ?.
6 is related to 29 in the same way 24 will be related to ? , It means we have to apply same mathematical operations to 24 to get ?.
So if we multiply 6 with 5 and then subtract 1 from result obtained in previous step like this ( 6 × 5 ) - 1 which will be equal to 29.
Same operation we have to apply to 24.
( 24 × 5 ) - 1 = 120 - 1 = 119
So Correct option is ( c ) 119Problem # 2
5 : 100 :: 7 : ?
(a) 135 (b) 91 (c) 196 (d) 49
Solution
Because in 1st case if we take Square of 5 then multiply it with 4 we shall have 100.
5² × 4 = 25 × 4 = 100
Same procedure will be applied in 3rd number by taking square of 7 then multiply it with 4
7² × 4 = 49 × 4 = 196
Correct option is ( c ) 196
Problem # 3
6 : 18 :: 4 : ?
(a)4 (b)6 (c)8 (d)10
Solution
Divide 6² by 2
6² ÷ 2 = 36 ÷ 2 = 18
Similarly divide 4² with 2
i.e. 4² ÷ 2 = 16 ÷2 = 8
Divide the square of 1st number by 2 to get 2nd number. Similarly take square of third number and then divide it by 2 to to get the number equal to? Since square of 4 is 16 then divided by 2 to get it.
Correct option is ( c ) 8
Divide 6² by 2
6² ÷ 2 = 36 ÷ 2 = 18
Similarly divide 4² with 2
i.e. 4² ÷ 2 = 16 ÷2 = 8
Divide the square of 1st number by 2 to get 2nd number. Similarly take square of third number and then divide it by 2 to to get the number equal to? Since square of 4 is 16 then divided by 2 to get it.
6² ÷ 2 = 36 ÷ 2 = 18
4² ÷ 2 = 16 ÷ 2 = 8
Correct option is ( c ) 8
Problem # 4
18 : 30 :: 36 : ?
(a)64 (b) 62 (c)54 (d) 66
Solution
(18 × 2 ) - 6 = 36 - 6 = 30
(36 × 2 ) - 6 = 72 - 6 = 66
correct option is ( d) 66
(18 × 2 ) - 6 = 36 - 6 = 30
(36 × 2 ) - 6 = 72 - 6 = 66
Multiply 1st number (18) with 2 and then subtract 6 from it
18 × 2 = 36 - 6 = 30
Similarly Multiply 3rd number ( 36 ) with 2 and then subtract 6 from it ,
36 × 2 = 72 - 6 = 66
Problem # 5
12 : 20 :: 30 : ?
(a)48 (b)42 (c)15 (d)35
Solution
Method 1
Split 12 = 3 × 4
Split 20 = 4 × 5,
Split 30 = 5 × 6,
Study these factors (3 , 4 ) , (4 ,5 ) ,(5 ,6 ) so next pair will be ( 6 , 7 ) , It means ? Will be replaced by the number 6 × 7 = 42
12 will be written as square of 3 plus 3 , 20 will be written as square of 4 plus 4 ,30 will be written as square of 5 plus 5, so next number will be written as square of 6 plus 6 which is equal to 42 , Therefore required option will be 42.
Or
Or
Make continuous factors 3 and 4 , 4 and 5 ,5 and 6 and 6 and 7 of 12, 20, 30 and 42 respectively
12 = 3 × 4 ,
20 = 4 × 5 ,
30 = 5 × 6 so
42 = 6 × 7
Add same number to its square to get next number
Or multiply next number to the number
9 = 3² + 3 ,
20 = 4² +4 ,
30 = 5² + 5
42 = 6² + 6
12 = 3 × 4 ,
20 = 4 × 5 ,
30 = 5 × 6 so
42 = 6 × 7
Add same number to its square to get next number
Or multiply next number to the number
9 = 3² + 3 ,
20 = 4² +4 ,
30 = 5² + 5
42 = 6² + 6
Correct option is ( b ) 42
Problem # 6
12 : 54 :: 15 : ?
(a)64 (b)69 (c)56 (d)67
Solution
{(1st number ) × 5} - 6 = 2nd number
(12 × 5) - 6 = 54
Multiply 3rd number with 5 then subtract 6 from it
{(3rd number ) × 5} - 6 = 4th number
(15 × 5) - 6 = 69
{(1st number ) × 5} - 6 = 2nd number
(12 × 5) - 6 = 54
Multiply 3rd number with 5 then subtract 6 from it
{(3rd number ) × 5} - 6 = 4th number
(15 × 5) - 6 = 69
Multiply first number ( 12 ) with 5 then subtract 6 from it to get 2nd number ( 54 ) , Similarly Multiply third number ( 15 ) with 5 then subtract 6 from it.
( 1 2 × 5 ) - 6 = 60 - 6 = 54
( 1 2 × 5 ) - 6 = 60 - 6 = 54
( 1 5 × 5 ) - 6 = 75 - 6 = 69
Correct option is ( b) 69
Problem # 7
6 : 5 :: 8 : ?
(a) 6 (b)10 (c) 2 (d)4
Solution
Add 4 to 1st number and divide it with 2 to get 2nd number( 6 + 4 )/2 = 5
Similarly in 2nd case Add 4 to 3rd number then divide the resultant with 2 to get 4th number
( 8 + 4 )/2 = 6
Correct option is ( a ) 6
Add 4 to 1st number and divide it with 2 to get 2nd number( 6 + 4 )/2 = 5
Similarly in 2nd case Add 4 to 3rd number then divide the resultant with 2 to get 4th number
( 8 + 4 )/2 = 6
Correct option is ( a ) 6
Problem # 8
29 : 319 :: 23 : ?
(a)115 (b)252 (c)151 (d)46
Solution
Add both the digits of first number i.e. 2 and 9 then multiply it with first number to get second number. similarly in the third number add both the digits I.e 2 and 3 and multiply it with 3rd number to get fourth number.
29 × ( 2 + 9 ) = 29 × 11 = 319
23 × ( 2 + 3 ) = 23 × 5 = 115
So 253 is the right option (a) 115
6 : 64 :: 11 : ?
(a) 127 (b) 124 (c) 144 (d) 169
Solution
Add 2 to 1st given number and take its Square to get 2nd number. Similarly add 2 to 3rd number and take it square to get fourth number.
(6 + 2 )² = 8² = 64
(11 + 2)² = 13² = 169
(6 + 2 )² = 8² = 64
(11 + 2)² = 13² = 169
Correct option is ( d ) 169
Problem # 10
36 : 50 :: 64 : ?
(a)70 (b) 82 (c)78 (d) 72
Solution
Take Square Root of 1st number and add 1 to it then add 1 to its Square to get 2nd number.
Similarly take square root of 64 add 1 to its square root and take it square and add 1 to get 4th number.
36 = 6² ---> (6 + 1)² + 1 = 50 ,
64 = 8² ------> ( 8 + 1)² + 1 = 82
√36 = 6 add 1 to it = 7 , square this number = 49 + 1 = 50
√64 = 8 add 1 to it = 9 , square this number = 81 + 1 = 82
So Correct option is ( b ) 82








No comments:
Post a Comment
Your valuable suggestions are always acceptable to us for betterment of this website