USING METHOD OF INTEGRATION ,HOW TO FIND AREA OF TRIANGLE BOUNDED BY THREE LINES

Using method of integration find the area of triangle,using the method of integration find the area of the region bounded by the lines,area of triangle by integration method 

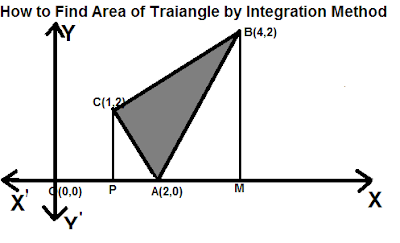
Using Method of Integration , How to find the area of triangle bounded by three lines 

2x + y = 0 , 3x - 2y = 6  and x - 3y  + 5 = 0

Solution

Given lines are

2x + y  = 4   --------  (1)
3x - 2y = 6  ---------  (2) and 
x - 3y  = -5  ---------  (3)


If these lines are intersecting then we have to find their coordinates of points of intersection .

To Find Coordinate of Point A

Multiply (1) by 2 and adding to (2) , we get
4x + 2y + 3x - 2y = 8 + 6
7x = 14 ⇒ x =2 
Putting x = 2 in (1) , we get 

Area under Curve

2(2) + y = 4
4 + y = 4 ⇒ y = 0
∴ (1) and (2) meets at point A(2,0).

To Find Coordinate of Point B

To find point of intersection (2) and (3);
Multiply (3) by -3 and adding to (2) , we get
3x - 2y -3x +9y  = 6 +15 
7y = 21  ⇒ y = 3 

Putting y = 3 in (3) , we get 
x-3(3)  = -5  
⇒ x = -5 +9  ⇒ x = 4 
∴ (2) and (3) meets at point B(4,3).

To Find Coordinate of Point C

To find point of intersection (1) and (3);
Multiply (1) by 3 and adding to (3) , we get
6x + 3y + x - 3y = 12 - 5 
7x = 7  ⇒ x =1 

Putting x = 1 in (1) , we get 
2(1) + y  = 4  
⇒ y =4 - 2  ⇒ y = 2 
∴ (1) and (3) meets at point C(1,2).

we get points of intersection of (1) and (2)  A(2,0),  points of intersection of (2) and (3) B(4,3) and points of intersection of (1) and (3) C(1,2).

Required Area = Shaded Area   =  Area DCBED - Area DCAD -Area ABEA

Also read my previous post  How to find area bounded by two circles 

For better understanding watch this video 

Conclusion 

 Thanks  for visiting this website and spending your precious time to read how to find area of triangle Using method of integration find the area of triangle,using the method of integration find the area of the region bounded by the lines,area of triangle by integration method.

If you are a mathematician Don't forget to visit my Mathematics You tube channel ,Mathematics Website and Mathematics Facebook Page , whose links are given below

Maths Website , Facebook Page and You Tube Channel

Read More

HOW TO SOLVE DETERMINANTS USING ELEMENTARY TRANSFORMATIONS

How to Prove Determinants using elementary transformations 

In this post we shall discuss Short trick of elementary transformation,Solving Determinants using elementary transformations,define elementary transformation, elementary transformation class 12, elementary row transformation questions.

By this method we have  to reduce maximum elements of specific Rows or column to zero, so that we can solve it easily

To solve the determinants using elementary transformations , Let us suppose L H S = △

As we can see that 'a' is common in 1st Row , 'b' is common in 2nd Row and 'c' is common in 3rd row ,
Therefore Taking a ,b ,c common from R_1  , R_2  , and  R₃       respectively

If we add R_1    to   R_2  and   R₁   to  R_{3   then we get zero in 1st column, so}  Operating  R_{1  →} R₁   + R₂   and    R_{3  →} R₁   + R₃  

_{As we have received maximum possible  zero in 1st column Therefore Expanding along} C₁  

△= (-a)×[(0)-(2c×2b)]
△ _{= abc{-a(-4bc)}}

△ = 4a²b²c² 

Hence the proof


Watch this video for Understanding Elementary transformations

PROBLEM

Proof:- Put L H S of determinant to Δ

Operating R₁ ➡️xR₁  , R₂ ➡️ yR₂ and R₃➡️ zR₃

Taking common xyz from C₃

Operating  R₂ ➡️ R₁ - R_2  and  R₃ ➡️ R₁ - R3

Expanding along  C₁ 

Δ = (x² - y² )( x³ - z³ ) - (x³ - y³ )(x² - z² )

Δ = (x- y )(x+ y)(x- z )( x² + z²  + xz ) - (x- y )( x² + y²  + xy ) (x - z )(x +z )

Δ = (x- y )(x- z )[(x+ y)( x² + z²  + xz ) -( x² + y²  + xy ) (x +z )]

Cancelling the same colour terms in the previous line ,then we have 

 Δ = (x- y )(x- z )[xz²   + yz² - y²x - y²z  ]

Arranging  terms in Squared Bracket  in such a way that the term containing z² must be at 1st and 3rd position and the term containing y² must be at 2nd and 4th position .

Δ = (x- y )(x- z )[(yz² - y²z) +( xz²  - y²x)]

Δ = (x- y )(x- z )[yz(z - y) + x(z²  - y²)]

Δ = (x- y )(x- z )[yz(z - y) + x(z - y)(z+ y)]

Δ = (x- y )(x- z )(z - y)[yz + x(z+ y)]

Δ = (x- y )(x- z )(z - y)[yz + xz+ xy]

Taking -1 common from (x- z )(z - y) in previous line ,

Δ = (x- y )(y- z )(z - x)[yz + xz+ xy]

Hence the   proof

Also Read   HOW TO FIND THE SLOPE OF LINE  AX + BY = C ,  SLOPE OF A  LINE

Final Words

Thanks for investing your precious time to read this post containing  Solving Determinants using elementary transformations,Short trick of elementary transformation  , elementary row transformation questions. If you liked it then share it with your near and dear ones to benefit them. we shall meet in next post with another beneficial article till then bye ,take care.......

If you are a mathematician Don't forget to visit my Mathematics You tube channel ,Mathematics Website and Mathematics Facebook Page , whose links are given below

Maths Website , Facebook Page and You Tube Channel

Read More

HOW TO FIND AREA BOUNDED BY TWO CIRCLES , INTEGRATION OF AREA UNDER CURVE

HOW TO FIND AREA OF TWO CIRCLES INTERSECTING EACH OTHERS,

Here we are going to discuss how to find common area of two circles which are overlapping or intersecting at two points with the help of an example

 Let us consider two circles whose equations are  given below
x² + y² =  1^{2                   .......................(1)}
( x - 1 )² + y² = 1²          .....................(2)

Let us draw these circles in coordinate planes, We can compare these equations with standard form of circle to find  the coordinate of  centre of both the circles are (0,0) and (1,0) respectively and radius of both the circles are 1.

If these two circles intersect with each other then we have to find their point/s of intersection.
To find points of intersection subtracting equation (1) from eq (2) , we get 

    ( X - 1 )² + Y² - X² - Y² =  1^2 - 1² 
⇒( X - 1 )²  - X² =  0
 ⇒X ²  +1 ²  - 2×(1)×(X) - X² =  0

⇒1 - 2X = 0

⇒ X = 1/2 ,
Now to find the values of y put the value of x in equation # 1

(1/2)² + Y² =  1²     
   Y² = 1- (1/4) = 3/4

   Y = 土⇃(3/4)
Therefore two points of intersection are B(1/2 , ⇃(3/4)) , C ( (1/2 , -⇃(3/4))

To understand better the solution of  this problem watch this  video 

Required area = shaded  Area , 

we can divide shaded area into four equal parts , As each parts is symmetrical , Therefore to find shaded area  it is sufficient to find the area of any one of four part and then then multiply it with 4.

Hence  Required area = 4 area OBLO = 4 Area BALB
To avoid tedious calculations choose 2nd part to integrate
I= Required area = 4 Area BALB    ------------- (3)

After simplification , we have

    ALSO READ        HOW TO INTEGRATE INTEGRAL WITH SQUARE ROOT IN NUMERATOR     

My previous post HOW TO FIND AREA OF THE CIRCLE  WHICH IS INTERIOR TO THE PARABOLA

  Final words 

Thanks for visiting this website and spending your valuable time to read this post regarding how to find area bounded by two circles .If you liked this post , do share it with your friends to benefit them also we shall meet in next post , till then bye and take care....

..

Read More

HOW TO FIND AREA OF THE CIRCLE WHICH IS INTERIOR TO THE PARABOLA

HOW TO FIND AREA OF THE CIRCLE  WHICH IS INTERIOR TO THE PARABOLA

Area Under Curves

Let  us write two equations of circle and parabola respectively
4x²+ 4y²  = 9 ------------------- (1) 

and x²      = 4y    -------------------(2)

Reducing (1) to standard form by dividing 4 .we get 
x²+ y²  = (3/2)²   
Ist of all draw figures of both the circle and the parabola in cartesian plane.

As it can be seen from figure both  curves intersect each other at two points say A and A' . 
Next we have to find these two coordinates points of intersection . Solving (1) and (2) to find the values of x and y 
Putting  the value of ' x² ' from (2) in (1) we get 

4(4y)+ 4y² = 9

16y + 4y² - 9 = 0

 4y² - 16y -9 = 0

        

 y = (-16+20)/8  and (-16-20)/8
y = 1/2  and -9/2

So Rejecting the -ve value of y ,because when we put negative value (-9/2) in eq (2) , we shall have two complex values of "x" which are not acceptable.
so only put positive value (1/2) of   'y'  in (2) we get two real values of  'x' such that    x= 土⇃2,
Now we can write coordinate M(⇃2,0) and N (-⇃2,0)

Required Area = Shaded area
                         = 2 × Area OBAO 
Note this step carefully ↑

                   

Multiplying every terms with 2 which is written at  beginning  of the previous line.

Putting the values of upper and lower limits of x

    ALSO READ        HOW TO INTEGRATE INTEGRAL WITH SQUARE ROOT IN NUMERATOR     

  Final words 

Thanks for visiting this website and spending your valuable time to read this post.If you liked this post , do share it with your friends to benefit them also we shall meet in next post , till then bye and take care......

Read More

HOW TO FIND THE ANGLE BETWEEN TWO LINES

HOW TO FIND THE ANGLE BETWEEN TWO LINES WHEN THE EQUATIONS OF GIVEN LINES ARE  IN CARTESIAN  FORMS

In this post we shall study How to find the angle between two lines ,angle between two lines vectors in Cartesian form, angle between two lines in 3d, angle between two lines calculator, angle between two lines coordinate geometry,derivation of angle between two lines

Problem 1

Consider two lines whose equations are given in cartesian form as

Note that direction Ratios of 1st line is (1 , 2 , 3) and the direction Ratios of 2nd line is (2 , 3 , 4).

Note that Direction Ratios of  any line are those numbers written is the denominator in standard form of the equation of the line

we know that if a_1,b_1,c_1   and    a_2,b_2,c_{2,  are Direction Ratios of line} L_{1  and Line} L<sub>2 .

If  θ be the angle between two lines ,Then this angle  can be formulated as follows</sub>

So putting the values of direction ratios of both the lines in (3) ;

we  get 

cos θ = [2 + 6 + 12]/sqrt[ 1+ 4+ 9]×sqrt[ 4 +9 +16 ]

cos θ = [20]/√[ 14×29 ];

cos θ = 10/√203

∴ θ = cos^-1 (10/√203)

Hence  cos^-1 (10/√203) is the angle between two lines

How to find angle between two  line in vector and cartesian   forms

 

Problem 2

Let us consider these two lines in cartesian form

Since these equations of lines are not in standard form  , in order to reduce these equations to standard form we shall have to make the coeffs of x, y , z unity.

In 1st part of equation (1) divide the num and den by 2 , and in 2nd part divide num and den by 3. 

Similarly divide 2nd part of  equation (2) by 5. we can rewrite these equations as follows

After cancellation and simplification , we get equations of lines in standard form

Here Direction Ratios of 1st Line are  (2,1 ,3)  and Direction Ratios of 2nd Line are (1 ,2 , 4) so using the formula

Putting the values of direction ratios of both the lines in above formula ,we get

cos θ = [2 + 2 + 12]/sqrt[ 4+ 1+ 9]×sqrt[ 1 +4 +16 ]

cos θ = [16]/sqrt[14×21];

cos θ = 16/√294

∴ θ = cos^-1 (16/√294)

Hence  cos^-1 (16/√294) is the angle between two lines

Problem 3

How to prove that the given lines are parallel to each others, 

To test whether given lines are parallel to each other , just check their direction ratios , id they are proportional to each others , then The given lines would be parallel to each other.

If the Direction ratios of 1st line is (1,2,5) and Direction ratios of 2nd  line is (2,4,10)  . If we take 2 common from direction ratios of 2nd lines then we have same direction Ratios as that of 1st line hence These lines are parallel o each other.

Problem 4

How to prove that the given lines are Perpendicular  to each others. If we calculate the angle between any two lines and it comes out to be 0 ( ZERO ) then  these would be perpendicular to each others.

E.g  If the D. R. of 1st line are ( 3, -1 , 3 )

And     D. R. of 2nd Line are  ( -2 ,3 ,3 ).

The cosine of angle between these  two line is zero

cos θ = 0

Then  θ = 90° 

⇒ Lines are Perpendicular to each others

Also Read previous posts 
How to Find perpendicular distance between skew lines

How to find slope of line ax+by=c


--------------------------------------------------------------------------------
To find the angle between two lines

x = y = z and

x = y = -z 


Want to check the solution of this problem ?

------------------------------------------------------------------------------
Thanks for your  precious time to  read this post regarding how to find the angle between two lines in Cartesian form.

Read More