Ten Most Important Reasoning Problems of Circles with Solutions, How to Solve Reasoning Circle Problems

Ten Most  Important Reasoning problems of circles with solutions. These types of problems are very helpful for cracking competitive exams like ssc cgl, ssc chsl and various Bank exams and many other similar exams .

Ten Most Important Reasoning Problems of Circles with Solutions.

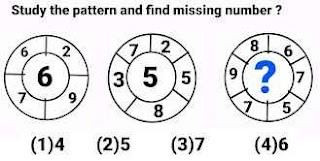
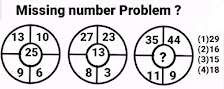
      Problem # 1

1st circle

Here 1st circle consist of four parts and if we divide sum of all the digits in its outer part with number of parts then result will be the middle number.

( 6 + 2 + 9 + 7  )/4 =  24/4 = 6 ( Middle number). 

2nd circle

2nd circle consist of five parts and if we divide sum of all the digits in its outer part with number of parts then result will be the middle number. 

( 7 + 2 + 5 + 8 + 3 )/5 = 25/5 =5 ( Middle number). 

3rd circle

3rd circle consist of six parts and if we divide sum of all the digits in its outer part with number of parts then result will be  the middle number. 

(  8 + 6 + 7 + 5 + 7 + 9 )/6 = 42/6 =7 ( Middle number). 

Hence option (3) is correct answer.

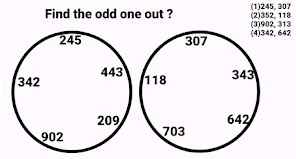
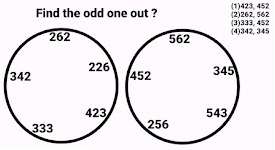
Problem # 2

This circle consists of four quadrants and every  quadrant consists of three numbers , And every quadrant have two numbers in outer part and  1 number in the inner part . To find the value of question mark  "?"  , we shall use two numbers which are in the outer part to calculate the value of the number which is in the inner part of every quadrant . 

1st Quadrant 

 8² ×  3⁴  =  64 × 81  = 5184

Now add all these digits  5 + 1 + 8 + 4 = 18

Now reversing the order of these digits obtained in  previous step

18   <----> 81 The number in the inner part

2nd Quadrant

  5² × 7⁴  =  25 ×  2401  = 60025

Now add all these digits  6 + 0 + 0 + 2  + 5  = 13

Now reversing the order of these digits obtained in  previous step

13   <---->  31 The number in the inner part

4th Quadrant

  8² ×  9⁴  =  64 × 6561  = 419904

Now add all these digits  4 + 1 + 9 + 9 + 0 + 4 = 27

Now reversing the order of these digits obtained in  previous step

27   <----> 72 The number in the inner part

3rd Quadrant

 7² × 7⁴  =  49 × 2401 = 117649

Now add all these digits  1 + 1 + 7 + 6 + 4 + 9  = 28

Now reversing the order of these digits obtained in  previous step

28   <----> 82 The number in the inner part

Option (1) is correct option.

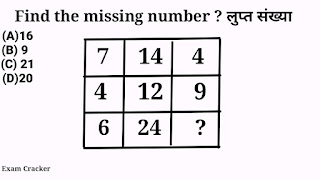
Problem # 3

This  circle has been divided into eight sectors. Every sector  consist of three numbers. To solve this problem multiply both the numbers  in any sector which are in the outer part and then add 1 to it ,the result so obtained is written in the inner part of the sector which is exactly opposite to this sector .Continuing in this manner  we shall have  all the numbers placed accordingly. .  Starting from the sector immediately to the right of question mark. 

( 2 × 4 ) + 1  =  9  ( In the inner part of 5th sector ) 

( 3 × 7 ) + 1 =  22  ( In the inner part of 6th sector )

( 1 × 6 ) + 1 =  7  ( In the inner part of  7th sector )

 ( 5 × 2 ) + 1  = 11  ( In the inner part of 8th sector )

( 3 × 4 ) + 1  =  13  ( In the inner part of 1st sector )

( 2 × 9 ) + 1  =  19  ( In the inner part of 2nd sector )

( 2 × 2 ) + 1  =  5  ( In the inner part of 3rd sector )

 ( ? × 3 ) + 1  =  25  ( In the inner part of 4th sector )

Now we have to find the value of  "?" like this 

( ? × 3 ) + 1  =  25

? × 3  =  25 - 1

? × 3  =  24

? = 24/3

? = 8 

Hence option (2)  is correct option. 

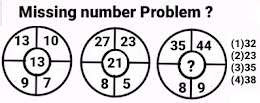
Problem # 4

1st Sector

(3 + 4 )² +  4² = 7² + 4² = 49 + 16 = 65 (The number is in circle opposite to 3 and 4)

2nd Sector

(7 + 2)² + 7² =  9² +  7² = 81 + 49 = 130 (The number is in circle opposite to 7 and 2)

3rd Sector

 (6 + 9)² +  (9)² = 15² +  9² =  225 + 81 = 306  (The number is in circle opposite to 6 and 9)

4th Sector

 (5 + 8 )² +  (8)² = 13² +  8² = 169 + 64 = 233 (The number is in circle opposite to 5 and 8)

Hence option (4) is right answer.

Problem # 5

All the Numbers except one in both the circles are written in same pattern . If we add all the three digits of any number in both the circles then we shall get same result except in one number in both the circles. And that different result will be the odd one out in this problem.

In 1st circle

The sum of digits of number 319 = 3 + 1 +  9  = 13  ( Odd one out)  

The sum of digits of number 441 = 4 + 4 + 1 = 9

The sum of digits of number 243 = 2 + 4 + 3 = 9

The sum of digits of number 612 =  6 + 1 + 2 = 9

The sum of digits of number 342 = 3 + 4 + 2 =  9  

In 2nd circle

The sum of digits of number 322 =  3 + 2 + 2 = 7

The sum of digits of number 313 =  3 + 1 + 3 = 7

The sum of digits of number 142 = 1 + 4 + 2 = 7 

The sum of digits of number 304 = 3 + 0 + 4 = 7

The sum of digits of number 349 = 3+ 4 + 9 = 16 ( Odd one out)

So from both these circles two results 13 and 16 of numbers 319 and 349  are different from other.

Hence option (4) is right answer.

Problem # 6

Since this circle consists of four quadrants and every quadrant consists of three numbers ,two numbers are in outer part and one number is in the inner part  ( triangle) . The  number which is in the inner part (triangle) can be found  using both the numbers which  are in the outer part of the same quadrant by using the following formula. 

(Difference of both  numbers  in the outer part) - ( difference of  both the digits in the triangular parts ) = 1 

1st Quadrants 

(12 - 8)  - ( 6 - 3 ) = 4 - 3 = 1

2nd Quadrants 

(4 - 3)  - ( 1 - 1  ) = 1 - 0 = 1

3rd Quadrants 

(9 - 6)  - ( 6- 4 ) = 3 - 2 = 1

4th Quadrants 

(12 - 10 )  - ( ? - ? ) = 2 - 1 = 1

If we put 43 in place of question mark only then difference of its digits  will be equal to 1 and in other cases the difference of digits is not equal to 1 which is necessarily required . 

So the correct option will be  (1) . 

 

 Problem # 7

All the Numbers except one in both the circles are written in same pattern . If we add all the three digits of any number in both the circles then we shall get same result except in one number in both the circles. And that different result will be the odd one out in this problem.

In 1st circle

The sum of digits of number 245 = 2 + 4 + 5 = 11

The sum of digits of number 443 = 4 + 4 + 3 = 11

The sum of digits of number 209 = 2 + 0 + 9 = 11

The sum of digits of number 902 = 9 + 0 + 2 = 11 

The sum of digits of number 342 = 3 + 4 + 2 =  9  ( Odd one out)

In 2nd circle

The sum of digits of number 307 =  3+ 0 + 7 = 10

The sum of digits of number 343 = 3 + 4 + 3 = 10

The sum of digits of number 642 = 6 + 4 + 2 = 12( Odd one out)

The sum of digits of number 703 = 7 + 0 + 3 = 10 

The sum of digits of number 118 = 1 + 1 + 8 = 10 

So from both these circles two results 9 and 12 of numbers 342 and 642  are different from other.

Hence option (4) is right answer.

Problem # 8

Starting clockwise take the difference of two consecutive numbers. 

3 - 1 = 2

6 - 3 = 3

11 - 6 = 5

18 - 11 = 7. 

Since all these resultant numbers are prime numbers so next prime number should be 11

Therefore  ? - 18 = 11

This implies ? = 29.

Hence option (D) is correct option. 

Problem # 9

Starting clockwise from the question mark i. e.  from 3 . These numbers 3, 5 ,7 ,11 ,13 are written in a pattern of prime number series. So so after 13  the next prime number will be 17. But 17 is not in the  given option. Hence this series will start from the prime number  prior to 3 . The number prior to 3 is 2 . Because smallest prime number is 2.

Hence pattern will be 2 , 3 , 5, 7 , 11, 13.

Therefore correct option will be (D) . 

Also read these posts on Reasoning

1.Reasoning for competive exams
2 .Box and circle reasoning
3 . Reasoning for bank exams
4. Ten Tricky logical reasoning
5. Missing  number series questions
6. Reasoning questions with answers
7. Circle Reasoning
8.  Box Problems
9. 15 Questions Circle Problems 
10. SSC CGL Reasoning

 

Problem # 10

This Circle consist of four quadrant and every quadrant consists of three numbers. Here the number in the outer part of every sector contribute to the number which is in the inner part of that sector . Starting from the sector which is below the question mark , out of these two numbers written in Outer part , the the number which is greater/maximum of these two is written in the inner part. 

In 1st Sector  

Max (14,17 ) = 17 , Here greater of the two numbers will be selected

In 2nd Sector  

Max (13,18) = 18, Here greater of the two numbers will be selected

In 3rd Sector  

Max (5 , 3 ) = 5, Here greater of the two numbers will be selected

In 4th Sector

  

Max ( 4, 8 ) = 8, Here greater of the two numbers will be selected

So option (2)  will be correct option

Also Reads these posts on Reasoning

Reasoning Problems #11  
Reasoning Analogy #10
Reasoning Questions #9    
Circle Reasoning #8
Ten Box-Problems #7   
Ten Reasoning problems #6
Ten-Important-Problem#5  
Ten--Tricky-Puzzles #4
Twelve-Figures-Problems#3   
Ten-Important-Reasoning#2     
Picture-Reasoning#1

Read More

Ten Most Important Box Problems in reasoning for Competitive Exams

Ten Most Important box problems in reasoning for SSC CGL,  SSC CHSL , RRB NTPC and other similar competitive exams. 

                                        Problem # 1

Multiplying the numbers in first column with numbers in second column , Its product must be equal to the multiplication of  numbers in third column and fourth column. 

Since all the numbers in third and fourth columns are given therefore we have to start from here.

 In 3rd  and 4th columns

1 × 24 = 24

8 ×  3  = 24

2 × 12 = 24

12 × 2 = 24

In 1st and 2nd columns 

6 × 4 = 24

4 × 6 = 24

3 × 8 = 24

 Similarly  ? × 1 = 24

Hence ? = 24

Hence option (D) is right option

               Problem # 2

Carefully study all the numbers in 1st row which are 3 ,8, 13, 18, ? , Now take the difference of two successive numbers, this is 5 in all the cases.

18 - 13 = 5 

13 - 8 = 5 

8 - 3 = 5

so  ? - 18 = 5

⇒ ? = 5 + 18 

⇒ ? = 23

Now study  all the numbers in 2nd row which are 2 ,11, 20, 29, ? , Now take the difference of two successive numbers, this is 9 in all the cases. 

Because  29 - 20 = 9

20 - 11 = 9

11 - 2 = 9

So ? - 29 = 9

⇒ ? = 9 + 29

⇒ ? =  38

Hence option (C) is right option

                                                                     Problem # 3

If we multiply 1st column with  the square root of 3rd column ,then we shall get the 2nd column.

7 × √4 = 7 × 2 = 14 (1st element in 2nd column)

4 × √9 = 4 × 3 = 12 ( 2nd element in 2nd column)

6  × √?   = 24 

 √?   =  4 

 √?   = 4 ,  squaring both sides

 ?    = 16   ( 3rd element in 2nd column)

Hence option (A) is right option

  Problem # 4

Taking the sum of squares of all the elements of 1st three rows to get the element in fourth row of respective column.

4² + 2² + 3² = 16 + 4 + 9 = 29          (Last number in 1st column)

 5² +  1² +  6² = 25 + 1 + 36 = 62        (Last number in 2nd column)

 1² + 2² + 3² = 1 + 4 + 9 = 14           (Last number in 3rd column)

5² + 5² + 2² = 25 + 25 + 4 = 54  ,The value of question Mark (Last number in 4th column)

Hence option (A) is right option

                                                                     Problem # 5

Look at the numbers in fourth row, these are large as compare to all other numbers.  All the numbers in fourth row are sum of 2nd row and product of 1st row and 3rd row .

( 7 × 3 )  +  ( 8 ) = 21 + 8 =  29  ( Last number in 1st column)

( 4 × 3 )  +  ( 7 ) = 12 + 7 =  19  ( Last number in 2nd column)

( 5 × ? )  +  ( 6 ) = 5? + 6 =  31  ( Last number in 3rd column)

⇒ 5? =  31 - 6 

⇒ 5? =  25

⇒ ? =  25/5

⇒ ? =  5

Hence option (D) is right option

                

 Problem # 6

 In this box problem the difference of the numbers in 1st and 2nd columns is equal to the number in 3rd column, while the sum of the numbers in 1st and 2nd columns is equal to the number in 4th column.

5  -  2 =  3 ( Number in 3rd column of 1st row )

6  -  3 =  3 ( Number in 3rd column of 2nd row  )

4  -  1 =  3 ( Number in 3rd column of 3rd row )

8  -  1 =  7 ( Number in 3rd column of 4th row )

5  +  2 =  7 ( Number in 4th column of 1st row )

6  +  3 =  9 ( Number in 4th column of 1st row  ) 

4  +  1 =  5 ( Number in 4th column of 1st row  )

8  +  1 =  9 ( Number in 4th column of 1st row   ) ,

 Therefore this will be the value of  question mark  "? "

Hence option (D) is right option

                                              Problem # 7

In this box problem every number in 2nd column is the sum of 1st and 3rd column.

481 + 365 = 846 

655 + 184 = 839

297 + 492 =  789 (The value of question mark)

Hence option (B) is right option

                                                        Problem # 8

In this box problem the difference of sum of last two columns and 1st two columns in every row is one.

( 10 + 5 ) - ( 6 + 8 )  = 15 - 14 = 1 ( 1st Row)

( 8 + 4 ) - ( 9 + 2 )   = 12  - 11 = 1  ( 2nd Row)

( 7 + 8 ) - ( 11 + 3 )  = 15 - 14 = 1 ( 3rd Row)

( ? + 2 ) - ( 5  + 8 )  = ( ? + 2 ) - ( 13) = 1 ( 4th Row)

So in order to get the difference equal to one we  have to put  ? = 12,

so that ( 12 + 2 ) - ( 5  + 8 )  = 14 -  13 = 1 ( 4th Row)

Hence option (B) is right option

Also read these posts on Reasoning

1.Reasoning for competive exams
2 .Box and circle reasoning
3 . Reasoning for bank exams
4. Ten Tricky logical reasoning
5. Missing  number series questions
6. Reasoning questions with answers
7. Circle Reasoning
8.  Box Problems
9. 15 Questions Circle Problems 
10.  SSC CGL Reasoning

                                                                      Problem # 9

In this box problem every number in fourth row of every column is the sum of  Multiplication of  all the numbers in every column and sum of all the numbers in every column. 

In 1st column ( 3 × 4 ×  5 ) + ( 3 + 4 + 5 ) = 60 + 12 = 72

In 2nd column ( 2 ×  5 × 6 ) + ( 2 + 5 + 6 ) = 60 +13 = 73

In 3rd column ( 5 × 9 × 1 ) + ( 5 + 9 + 1 ) = 45 + 15 = 60 ( The value of question mark )

Hence option (D) is right option

                                              Problem # 10

Multiplying the numbers in first column with numbers in second column , Its product must be equal to the multiplication of  numbers in third column and fourth column. 

Since all the numbers in 1st and  2nd columns are given therefore we have to start from here.

 In 1st and 2nd columns 

6  ×  8 =  48

4 ×  12  = 48

3 ×  16  = 48

8  ×  6  =  48

 In 3rd  and 4th columns

1 × 48 = 48

8  ×  6  =  48

16 × 3 =  48

 Similarly multiplication of  2  × ? = 48

Hence ? =  24

Hence option (D) is right option

Also reads these posts on Reasoning
ReasoningProblems #11  
ReasoningAnalogy #10
Reasoning Questions #9    
Circle Reasoning #8
TenBox-Problems #7   
TenReasoning problems #6
Ten-Important-Problem#5  
Ten--Tricky-Puzzles #4
Twelve-Figures-Problems#3   
Ten-Important-Reasoning#2     
Picture-Reasoning#1

Read More

15 Most Important Questions of Reasoning in Circle Problems

15 Missing term in circle reasoning with solution have been discussed in this post . These types of problems are very helpful for cracking competitive exams like ssc cgl, ssc chsl , RRB NTPC, Group D and various Bank exams and many other similar exams.

15 Most Important circle Problems of Reasoning for different competitive Exams

Problem # 1

Add all the numbers in every circle except middle number then divide it with 3 to get middle number.

{ 13 + 10 + 7 + 9  } / 3 = 39 / 3 = 13 ( Middle number in 1st picture)

{ 27 + 23 + 5 + 8  } / 3 = 63 / 3 = 21 ( Middle number in 2nd picture)

{ 35 + 44 + 9 + 8  } / 3 = 96 / 3 = 32 ( Middle number in 3rd picture)

Hence option (1) is right answer.

Problem # 2

Multiply both the numbers in the second line then divide it with difference of the numbers in the 1st line of every figure. In last step add "7" to it to get the number in the middle of every figure.

{ ( 9 * 6 ) / ( 13 -10 ) } + 7 = ( 54 / 3 )  + 7 =  18 + 7 = 25 ( Middle number in 1st picture )

{ ( 8 * 3 ) / ( 27 - 23) } + 7 = ( 24 / 4 )  + 7 =  6 + 7 =  13 ( Middle number in 2nd picture )

{ ( 11 * 9 ) / ( 44 - 35 ) } + 7 = ( 99 / 9 )  + 7 =  11 + 7 = 18 ( Middle number in 3rd picture ).

Hence option (4) is right answer.

Problem # 3

This figure consist of four sectors and every sector consists of three numbers. Every number in the inner part of each sector is H C F ( Highest Common Factor ) of two other numbers, which are in outer part of each sector.

HCF of 12 and 27 = 3
HCF of 16 and 24 = 8
HCF of 2 and 3 = 1                            

Similarly HCF of 4 and 8  = 4  ( The value of question mark )

Hence option (1) is right answer.

Problem # 4

This figure consist of four sectors and every sector consists of three numbers. Every number in the inner part of each sector is HCF ( Highest Common Factor ) of two other numbers which are in outer part of each sector.

  H C F of 12 and 15 = 3 

   H C F of   6 and 4 = 2 

   H C F of 3 and  2 = 1 

  H C F of 12 and 6 = 6 ( The value of question mark)

Hence option (4) is right answer.

Problem # 5

This figure consist of four sectors and every sector consists of three numbers. Every number in  the inner part  is LCM ( Lowest Common Multiple ) of two other numbers which are in outer part of each sector.

LCM of 12 and 15 = 60 

LCM  of  9 and 6 = 18

LCM  of  3 and 2 = 6 

LCM of 8 and 6 = 24  (The value of question mark)

Hence option (4) is right answer.

 Problem # 6

All the Numbers except one in both the circles are written in same pattern . If we multiply all the three digits of any number in both the circles then we shall get same result except in one number in both the circles. And that different result will be the odd one out in this problem.

In 1st circle

The product of digits of number 262 = 2 * 6 * 2 = 24

The product of digits of number 226 = 2 * 2 * 6 = 24

The product of digits of number 423 = 4 * 2 * 3 = 24

The product of digits of number 333 = 3 * 3 * 3 = 27 ( Odd one out)

The product of digits of number 342 = 3 * 4 * 2 = 24

In 2nd circle

The product of digits of number 562 = 5 * 6 * 2 = 60

The product of digits of number 345 = 3 * 4 * 5 = 60

The product of digits of number 543 = 5 * 4 * 3 = 60

The product of digits of number 256 = 2 * 5 * 6 = 60 

The product of digits of number 452 = 4 * 5 * 2 = 40 ( Odd one out)

So from both these circles two results 27 and 40 of numbers 333 and 452 are different from other.

Hence option (3) is right answer.

Problem # 7

All the Numbers except one in both the circles are written in same pattern . If we add all the three digits of any number in both the circles then we shall get same result except in one number in both the circles. And that different result will be the odd one out in this problem.

In 1st circle

The sum of digits of number 163 = 1+ 6 + 3 = 10

The sum of digits of number 145 = 1 + 4 + 5 = 10

The sum of digits of number 334 = 3 + 3 + 4 = 10

The sum of digits of number 441 = 4 + 4 + 1 = 9  ( Odd one out)

The sum of digits of number 343 = 3 + 4 + 3  = 10

In 2nd circle

The sum of digits of number 414 =  4 + 1 + 4 =  9

The sum of digits of number 333 = 3 +  3 + 3 =  9

The sum of digits of number 444 = 4 + 4  + 4 =  12  ( Odd one out). 

The sum of digits of number 504 = 5 + 0 + 4 =  9 

The sum of digits of number 108 = 1 + 0 + 8 = 9 

So from both these circles two results 9 and 12 of numbers 441 and 444 are different from other.

Hence option (2) is correct answer.

 

Problem # 8

In this circle all the numbers are written in a line and the sum of each line is 25. Starting from the line which is right side of ? ( Question mark),

 4 + 11 + 3 + 7 = 25

10 + 1 + 9 + 5 = 25

11 + 6 + 2 + 6 = 25

? + 5 + 5 + 8  = 25

⇒   ? + 18 = 25 

? = 25 - 18 = 7

Hence option (1) is correct answer

Also read these posts on Reasoning

1.Reasoning for competive exams
2 .Box and circle reasoning
3 . Reasoning for bank exams
4. Ten Tricky logical reasoning
5. Missing  number series questions
6. Reasoning questions with answers
7. Circle Reasoning
8.  Box Problems
9. 15 Questions Circle Problems 
10.  SSC CGL Reasoning

 Problem # 9

Here 1st circle consist of four numbers and if we divide sum of all the digits in its outer part with total numbers then result will be the middle number.

( 3 + 8 + 7 + 2  )/4 =  20/4 = 5 ( Middle number)

2nd circle consist of five numbers and if we divide sum of all the digits in its outer part with total numbers then result will be the middle number. 

( 6 + 4 + 8 + 5 + 7 )/5 = 30/5 =6 ( Middle number)

3rd circle consist of six numbers and if we divide sum of all the digits in its outer part with total numbers then result will be  the middle number. 

(  9 + 7 + 8 + 9 + 7 + 8   )/6 = 48/6 =8 ( Middle number)

Hence option (2) is correct answer

Problem # 10

This figure consist of four sectors and every sector consists of three numbers. Every number in  the inner part  is cube of difference of two other numbers which are in outer part of each sector.

Cube of ( 8 - 5 )  =  3³ = 27  

Cube of  ( 23 - 19 ) = 4³ = 64

Cube of ( 3 - 2 ) =   1³ = 1

Cube of  ( 26 - 24 ) = 2³ = 8 ( The value of question mark)

Hence option (4) is correct answer

Problem # 11

This figure consist of four sectors and every sector consists of three numbers. Every number in  the inner part  is  Four times of square of difference of two other numbers which are in outer part of each sector.

4 × Square of ( 11 - 8 )  =  4 × (3)²  =  4 × 9 = 36  

4 × Square of ( 5 - 5 )  =  4 × (0)²  =  4 ×  =  0 

4 × Square of ( 7 - 3 )  =  4 × (4)²  =  4 × 16 = 64  

4 × Square of ( 8 - 2 )  =  4 × (6)²  =  4 × 36 = 144 ( The value of question mark)

Hence option (1) is correct answer

Problem # 12

This figure consist of four sectors and every sector consists of three numbers. Every number in  the inner part  is cube of difference of two other numbers which are in outer part of each sector.

Cube of (11 - 8 )  =  3³ = 27  

Cube of  ( 5 - 4 ) =   1³ = 1

Cube of ( 7 - 3 ) =    4³ = 64

Cube of  ( 8- 2 )  =  6³ = 216 ( The value of question mark)

Hence option (1) is correct answer

Problem # 13

This figure consist of four sectors and every sector consists of three numbers. Every number in  the inner part  is five times of  product of two other numbers which are in outer part of each sector.

( 6 × 3 ) × 5 = 18 × 5 = 90

( 2 × 3 ) × 5 =  6  × 5 = 30

( 4 × 2 ) × 5 =  8  × 5 = 40

( 5 × 4 ) × 5 = 20 × 5 = 100 ( The value of question marks ) . 

Hence option (1) is right answer.

Problem # 14

This figure consist of four sectors and every sector consists of three numbers. Every number in  the inner part  is the sum  of  two other numbers which are in outer part of opposite sector to these numbers.

 24 + 11 = 35 ( The number opposite to both 24 and 11 ) . 

13 + 26 = 39 ( The number opposite to both 246and 13 ) . 

9  +  25 = 34 ( The number opposite to both 9 and 25 ) . 

7 + ? = 35 ( The number opposite to both 7 and ? ) . 

So if we put ? = 28 then we shall have total equal to 35.

Hence option (2) is right answer

Problem # 15

All the Numbers except one in both the circles are written in same pattern . If we multiply all the three digits of any number in both the circles then we shall get same result except in one number in both the circles. And that different result will be the odd one out in this problem.

In 1st circle

The product of digits of number 145 = 1 *  4 *  5  = 20

The product of digits of number 451 = 4 * 5 * 1 =  20

The product of digits of number 225  = 2 * 2 * 5 = 20

The product of digits of number 541 = 5 * 4 * 1  = 20 

The product of digits of number 255 = 2 * 5 * 5 = 50 (Odd one out)

In 2nd circle

The product of digits of number 414 = 4 * 1 * 4  = 16

The product of digits of number 444 = 4 *  4  * 4  = 64  (Odd one out)

The product of digits of number 224 = 2 * 2  * 4  = 16

The product of digits of number 441 = 4  * 4 * 1  = 16 

The product of digits of number 128 = 1 * 2 * 8  =  16  

So from both these circles two results 50 and 64 of numbers 255 and 444 are different from other.

Hence option (3) is right answer.

Also Reads following posts on Reasoning

ReasoningProblems #11  
ReasoningAnalogy #10
Reasoning Questions #9    
Circle Reasoning #8
TenBox-Problems #7   
TenReasoning problems #6
Ten-Important-Problem#5  
Ten--Tricky-Puzzles #4
Twelve-Figures-Problems#3   
Ten-Important-Reasoning#2     
Picture-Reasonin#1

Read More