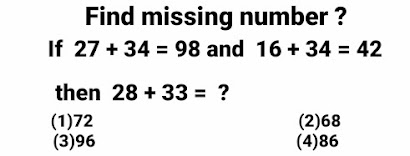Missing number series questions and answers, Missing Number Series Questions for SBI Clerk

Ten most important Missing number series questions and answers, Number Series Questions for SBI Clerk ,SBI PO with solution , for SSC GL , SSC CHSL , RRB NTPC and other competitive Exams  have been discussed in this post.  These types of series questions are asked in many other competitive exams like SI, CPO and various entrance exams. 

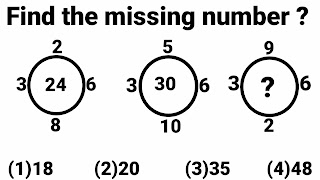
Problem #1

This problem is a series problem where we have to find the value of question mark using all its previous terms by analysing the trend of all its terms whether they are in increasing order or decreasing order or in any other format.

Formula :- 

Every term is the product of its two preceding terms.

1st number = 3
2nd number = 3

3rd number = (1st number )×( 2nd number ) = 3 × 3 = 9

4th number = ( 2nd number )×( 3rd number ) = 3 × 9 = 27

5th number = ( 3rd number )×( 4th number ) = 9 × 27 = 243

6th number = ( 4th number )×( 5th number ) = 243 × 27 = 6561

Option (4)6561 is correct option 

Problem #2

This problem is a series problem where we have to find the value of question mark using all its previous terms means by analysing the trend of all its terms whether they are in increasing order or decreasing order. This reasoning problem can be solved by two method . we shall discuss both these methods one by one.

Method #1 

35 -15 = 20 (Here difference of 1st and 2nd term is 20)

63 - 35 = 28 (Here difference of 2nd and 3rd term is 20 + 8 = 28, i.e. 8 more than previous difference)

99 - 63 = 36 (Here difference of  3rd and 4th term is 28 + 8 = 36, i.e. 8 more than previous difference)

143 - 99 = 44 (Here difference of  4th and  5th term is 36 + 8 = 44, i.e. 8 more than previous difference)

? - 143 = 52 (Here difference of  5th and  6th term must be  44 + 8 = 52, i.e. 8 more than previous difference)

⇒  ? = 52 + 143 = 195  

Option (2)195 is correct option 

Method #2

All these terms are one less than perfect squares of even numbers. 

4² - 1 = 16 -1 = 15 

6² - 1 = 36 -1 = 35 

8² - 1 = 64 -1 = 63 

10² - 1 = 100 -1 = 99

12² - 1 = 144 -1 = 143

14² - 1 = 196 -1 = 195 

Option (2)195 is correct option

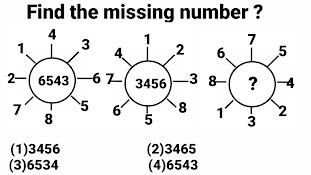
Problem #3

This reasoning problem is a series problem where we have to find the value of question mark using all its previous terms means by analysing the trend of all its terms whether they are in increasing order or decreasing order. The value of question mark can be found using the difference of two of its previous terms. And after analysing  the difference so obtained we can find the value of question mark.

13 - 6 = 7

27 - 13 = 14

55 - 27 = 28

111 - 55 = 56

?  - 111 = 112

⇒ ? = 112 + 111

⇒ ? = 223

Option (3)223 is correct option

Problem #4

This reasoning problem is a series problem where we have to find the value of question mark using all its previous terms means by analysing the trend of all its terms whether they are in increasing order or decreasing order. 

1st number = 8

2nd number = (1st number  × 1 ) + 1 

( 8 × 1 ) + 1 = 8 + 1 = 9

3rd number = ( 2nd number × 2 ) + 2 

( 9 × 2 ) + 2 = 18 + 2 = 20

4th number = ( 3rd number × 3 ) + 3 

( 20 × 3 ) + 3 = 60 + 3 = 63

5th number = ( 4th number × 4 ) + 4

( 63 × 4 ) + 4 = 252 + 4 = 256

6th number = ( 5th number × 5 ) + 5

( 256 × 5 ) + 5 = 1280 + 5 = 1285

Option (2)1285 is correct option.

Problem #5

These numbers are in binary number system. So 1st of all convert these numbers to decimal number system. 

11(binary number system) = 3 (decimal number system) . 

100(binary number system) = 4 (decimal number system) 

111(binary number system) = 5  (decimal number system) 

Similarly 

1000(binary number system) = 6 (decimal number system) 

 Option (2)1000 is correct option

Problem #6

This reasoning problem is a series problem where we have to find the value of question mark using all its previous terms means by analysing the trend of all its terms whether they are in increasing order or decreasing order.

(9 × 1) - 2 = 9 - 2 = 7 ( 2nd Number )
(7 × 2) - 3 = 14 - 3 = 11 ( 3rd Number )
(11 × 3) - 4 = 33 - 4 = 29 ( 4th Number )
(29 × 4) - 5 = 116 - 5 = 111 ( 5th Number )
(111 × 5) - 6 = 555 - 6 = 549 ( 6th Number ) = value of question mark

Option (4)549 is correct option

Also Reads these articles

 

 Latest-Questions-of-missing-numbers 

Maths logical reasoning Q & A

Reasoning for competive exams
Box and circle reasoning
 Reasoning for bank exams
Ten Tricky logical reasoning

 

Problem #7

This reasoning problem is a series problem where we have to find the value of question mark using all its previous terms means by analysing the trend of all its terms whether they are in increasing order or decreasing order. 

57 - 56 = 1= 1²

56- 55 = 1 = 1³

55 - 51 = 4 = 2²

51 - 43 = 8 = 2³

43 - 34 = 9 = 3²

Similarly ? - 34 = 4² = 16

⇒? - 34 = 16

⇒? = 16 + 34

⇒? = 50

Option (2)50 is correct option

Problem #8

This reasoning problem is a series problem where we have to find the value of question mark using all its previous terms means by analysing the trend of all its terms whether they are in increasing order or decreasing order. The value of question mark can be found using the difference of two of its previous terms. And after analysing  the difference so obtained we can find the value of question mark.

336 - 224 = 112

224 - 168 = 56

168  - 140 = 28

140 - 126 = 14

Similarly continuing in the same pattern 

126 - ? = 7 

⇒-? = 7 - 126

⇒? = -7  + 126

⇒? = 119

Option (3)119 is correct option.

Problem #9

Formula :- Sum of both digits of each numbers are same except one.

Sum of both the digits of 30 ⇒ 3 + 0 = 3

Sum of both the digits of  27 ⇒ 2 + 7 = 9

Sum of both the digits of 36 ⇒ 3 + 6 = 9

Sum of both the digits of  45 ⇒ 4 + 5 = 9

Sum of both the digits of  72 ⇒ 7 + 2 = 9

All the numbers except 30 have have it's digits sum equal to 9. Only the number 30 have it's digits sum equal to 3 . So 30 is the odd number and it must be out. 

Option (2)30 is correct option

Also Reads these articles

 Missing  number series questions

Reasoning questions with answers
Circle Reasoning
 Box Problems
15 Questions Circle Problems 
 SSC CGL Reasoning

Problem #10

Separating these given numbers to two series by picking odd number and even number position. 

1st series 

5 , 7 , 10 , 14 , 

Difference 2 , 3 , 4 , 5

2nd series

6 , 8 , 11 , ? 

Difference 2, 3, 4

Hence ? = 15

Option (3)15 is correct option.

Your Queries

(By Jai Singh Nayak)

Missing number in series 
1,  6,  26, _____ , 426

  Formula 

  Next Term =  (4 × Previous Term ) + 2.
  1st Term = 1
  2nd Term =  (4 × 1st Term ) + 2
  2nd Term =  (4 × 1 ) + 2
                     = 4 + 2
                     = 6
                     
  3rd Term =  (4 × 2nd Term ) + 2
  3rd Term =  (4 × 6 ) + 2
                   = 24 + 2
                   = 26
 4th Term =  (4 × 3rd Term ) + 2
 4th Term =  (4 × 26 ) + 2
                  = 104 + 2
                  = 106
 5th Term =  (4 × 4th  Term ) + 2
 5th Term =  (4 × 106 ) + 2
                  = 424 + 2
                  = 426
 6th Term =  (4 × 5th Term ) + 2
 6th Term =  (4 × 426 ) + 2
                  = 1704 + 2
                  = 1706
  7th Term =  (4 × 6th Term ) + 2
  7th Term =  (4 × 1706 ) + 2
                    = 6824 + 2
                    = 6826.

By MD Kaif

Complete the series

1, 3, 4, 8, 15 , 27, ?

Formula

To find any term after 3rd Term = Sum of its previous three terms . 

4th term = 1st term + 2nd term + 3rd term = 1 + 3 + 4 = 8 

5th term = 2nd term + 3rd term + 4th term = 3 + 4 + 8 = 15

6th term = 3rd term + 4th term + 5th term = 4 + 8 + 15 = 27

7th term = 4th term + 5th term + 6th term = 8 + 15 + 27 = 50 ( the value of question mark ).

By Shavnam Sharma

Complete the series

343, 729, 1331, 2197, ?  

  Formula 

  Any Term = (n)³ ,where n is odd number greater than equal to 7

  Or

  Any Term = (2n+1)³ ,where n is odd number greater than equal to 3.

That means every term has been expressed as the cube of some odd numbers.

  

  1st Term = 343 = 7³

  2nd Term = 729 = 9³

                     

  3rd Term = 1331 = 11³

   

 4th Term = 2197 = 13³

  

 5th Term = 15³ = 3375

 

 So the value of question mark = 3375

 Similarly if you want to find out the value of next terms then it will be

  

6th Term = 17³ = 4913

7th Term = 19³ = 6859

8th Term = 21³ = 9261

9th Term = 23³ = 12167

10th Term = 25³ = 15625

                                                       
Ten most important Missing number series questions and answers, Number Series Questions for SBI Clerk ,SBI PO with solution  for SSC GL , SSC CHSL , RRB NTPC and other competitive Exams were discussed in this post.  comment your valuable suggestions regarding this post and for further improvement.         

Read More

Ten Missing number questions with solutions for ssc cgl exam

Ten Missing number questions with solutions for ssc cgl exam are discussed in this post . These questions of reasoning in latest reasoning questions with answers are very very important for upcoming competitive exams  like Bank PO , SSC CGL etc . So let us start solving and understanding these Maths logical reasoning questions with answers.

Problem # 1

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- 3 times the sum of four numbers around the circle

3 (4 + 3 + 2  + 1 ) = 3 × 10 = 30  (  middle number in 1st figure  ) 

3 ( 5 + 4  + 3 + 2  ) = 3 × 14 = 42  ( middle number in 2nd figure  ) 

3 ( 6 + 5 + 4 + 3 ) = 3 × 18 = 54  (middle number in 3rd figure) 

Option (1)54 is correct option

Problem # 2

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- Left number + ( Product of remaining three numbers ) = Middle number

3 + ( 3 × 4 × 5 ) = 3 + 60 =  63 ( middle number in 1st figure  ) 

6 + ( 4 × 5 × 3) = 6 + 60 = 66 ( middle number in 1st figure  ) 

6 + ( 7 × 3 × 5 ) = 6 + 105 =  111 ( middle number in 1st figure  ) 

Option (1)71 is correct option

Problem # 3

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- Sum of squares of four numbers around the circle is  = Middle number

1² + 4² + 3² + 2² = 1 + 16 + 9 + 4 = 30 ( middle number in 1st figure ) 

2² + 5² + 4² + 3² = 4 + 25 + 16 + 9 = 54 ( middle number in 2nd figure ) 

3² + 6² + 5² + 4² = 9 + 36 + 25 + 16 = 86 ( middle number in 3rd figure ) 

Option (1)86 is correct option

Problem # 4

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- Product of cube root of four numbers around the circle  = Middle number

∛8 ×∛64 ×∛27 ×∛125 = 2 × 4 × 3 × 5 = 120 ( Middle number in 1st circle) 

∛1 ×∛216 ×∛64 ×∛27 = 1 × 6 × 4 × 3 = 72 ( Middle number in 2nd circle) 

∛8 ×∛343 ×∛125 ×∛1000 = 2 × 7 × 5 × 10 = 700 ( Middle number in 3rd circle) = The value of question mark, But 700 is not in any of three options. Therefore 

Option (4)NOA is correct option

 Problem # 5

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- Sum of cube root of four numbers around the circle = Middle number

∛8 + ∛64 + ∛27 + ∛125 = 2 + 4 + 3 + 5 = 14 ( Middle number in 1st circle) 

∛1 + ∛216 + ∛64 + ∛27 = 1 + 6 + 4 + 3 = 14 ( Middle number in 2nd circle) 

∛8 + ∛343  + ∛125 + ∛1000 = 2 + 7 + 5 + 10 = 24 ( Middle number in 3rd circle) = The value of question mark. Therefore

Option (4)24 is correct option

Problem # 6

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- Sum of square root of four numbers around the circle   = Middle number

√4+ √16 + √9 + √1 = 2 + 4 + 3 + 1 = 10 ( Middle number in 1st circle)

 √64+ √25 + √16 + √36 = 8 + 5 + 4 + 6 = 23 ( Middle number in 2nd circle)

 √49+ √256 + √144 + √289 = 7 + 16 + 12 + 17 = 52 ( Middle number in 3rd circle)

Option (2)52 is correct option

Problem # 7

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- Product of square root of four numbers around the circle  = Middle number

√4× √16 × √9 × √1 = 2 × 4 × 3 × 1 = 24 ( Middle number in 1st circle)

 √64+ √25 + √16 + √36 = 8 × 5 × 4 × 6 = 960 ( Middle number in 2nd circle)

 √9 + √100+ √16 + √121 = 3 × 10 × 4 × 11 = 1320 ( Middle number in 3rd circle)

Option (2)1320 is correct option

Problem # 8

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- Sum of square root of cube root of four numbers around the circle   = Middle number

(∛8)² + (∛125)² + (∛27)² + (∛64)² = 2² + 5² + 3² + 4² =  4 + 25 + 9 + 16 = 54 ( Middle number in 1st circle) 

(∛1) ² + (∛27)² + (∛64)² + (∛216)²= 1² + 3² + 4² + 6² = 1 + 9 + 16 + 36 = 62  ( Middle number in 2nd circle) 

(∛8) ² + (∛343)² + (∛125)² + (∛100)²= 2² + 7² + 5² + 10² = 4 + 49 + 25 + 100 = 178 ( Middle number in 3rd circle)  = The value of question mark. Therefore

Option (4)178 is correct option

Also Read these articles

Latest-Questions-of-missing-numbers 

Maths logical reasoning Q & A

Reasoning for competive exams

Box and circle reasoning

 Reasoning for bank exams

Ten Tricky logical reasoning

Missing  number series questions

Reasoning questions with answers

Circle Reasoning

 Box Problems

15 Questions Circle Problems 

Problem # 9

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- Highest common factor of four numbers around the circle  = Middle number

HCF of  9, 12, 6 and 18 = 3

HCF of 30, 50, 60 and 20 = 10

HCF of  3, 5, 6 and 2 = 1 = The value of question mark. 

Option (4)NOA is correct option

Problem # 10

Exam Cracker

There are three circles in this reasoning problem and every circle have five numbers associated to it. Out of these five numbers four are placed around the circle and fifth is in the centre of the circle. In the 3rd circle the value of question mark will be calculated using all the four associated numbers using the same formula which have been used in the 1st and 2nd circle to calculate the value of middle number.

Formula :- Least common multiple of four numbers around the circle  = Middle number

LCM of  3, 2, 6 and 8 = 24

LCM of 3, 5, 6 and 10 = 30

LCM of  3, 9, 6 and 2 = 18 = The value of question mark. 

Option (1)18 is correct option

Comment your valuable suggestion regarding the post "most important Reasoning questions with answers" which includes reasoning for competitive exams, circle problems, box problems, circle problems and triangles problems  for competitive exams like SSC CGL ,SSC CHSL ,CPO ,Bank exams and RRB NTPC etc which were explained in this post.

Read More

Ten Questions of number analogy for competitive exams

Ten Questions of missing numbers for competitive exams and missing number in reasoning for competitive exams like Bank PO, Bank clerk, SSC CGL, ssc chsl, RRB NTPC , group D etc have been discussed in this post .

Ten Questions of number analogy for competitive exams

Problem # 1

To find the value of question mark, 1st add both the digits of  1st number and 2nd number and then multiply the results so obtained. 

Formula :- (Sum of  both digits of 1st number) × ( Sum of  both digits of 2nd numbers) .

(3 + 5 ) × (6 + 5) = 8 × 11 = 88 ( It is given) 

(3 + 6 ) × (7 + 4) = 9 × 11 = 99 ( It is given)

(4 + 4 ) × (5 + 5) = 8 × 10 = 80 ( The value of question mark )

Option (4) 80 is correct option

Problem # 2

 To find the value of question mark, 1st multiply both the digits of  1st number and  add both the digits of 2nd number  and then multiply the results so obtained. 

Formula :- (Product of  both digits of 1st number) × (Sum of  both digits of 2nd number).

(2 × 7) × ( 3 + 4 ) = 14 × 7 = 98

(1 × 6 ) × ( 3 + 4 ) =  6 × 7 = 42

(2 × 8 ) × ( 3 + 3 ) = 16 × 6 = 96

Option (3)96 is correct option

Problem # 3

 In this problem all the three given numbers are nearer to the cube of any number. Hence all the given numbers can be written as the cube of some number in addition to one more mathematical operation. If we look carefully then all these given numbers can be written as the sum of a number and cube of the same number.

Formula :-  a³ + a

 8³ + 8 = 512 + 8 = 520 (1st number in given problem)

 9³ + 9 = 729 + 9 = 738 (2nd number in given problem)

 6³ + 6 = 216 + 6 = 222 (3rd number in given problem)

 7³ + 7 = 343 + 7 = 350 (4th number in given problem) and this will be the value of question mark. 

Option (4)350 is correct option

Problem # 4

 In this reasoning problem 1st number (167) is associated to 43 with the help of any rule , in the same rule we have to associate 245 to a number out of four given option.

Look carefully the given numbers consists of three digits. These three digits can be utilised with the help a formula given below.

Formula :- (Left most digit) +{middle digit × right most digit}

{ 1 + ( 6 × 7) } = 1 + 42  = 43

{ 2 + ( 4 × 5) } = 2 + 20  = 22

Option (1)22 is correct option

Problem # 5

Exam Cracker

In this reasoning problem 1st number (167) is associated to 112 with the help of some rule , With the help of same rule we have to associate 452 to a number out of four given option. Also in this problem all the  numbers consists of three digits. These three digits can be used to find the value of question mark with the help a formula given below.

Formula :- (Two Left most digits  ×  right most digit}.

In this problem both the left most digits can be taken as single unit to multiply with the 3rd number. 

{ 16  × 7 } = 112

{ 45 × 2 } =  90 = ? ( The value of question mark ) 

Option (4)90 is correct option

Problem # 6

 In this reasoning problem 1st number (824) is associated to 3 with the help of any rule , in the same way we have to associate 999 to a number out of four given option. All the three digits of given numbers can be utilised with the help a formula given below.

Formula :- (Two Right most digits  ÷  Left most digit}

In this problem both the two right most digits can be taken as single unit to get divided with the 3rd number. 

24 ÷ 8 = 3

99 ÷ 9 = 11= ? ( The value of question mark) 

Option (2)11 is correct option

Problem # 7

 This reasoning problem also consists of three digits .In this problem we have to transform 124 to 21 using a rule in the same way we have to change 631 to a number out four given options. To solve this problem means to get the 2nd number from 1st number ,take the squares of all the digits of 1st number and add all the three results so obtained.

Formula :- (1st digit)²  +  (2nd digit)² +  (3rd digit)² 

 1² +  2² +  4²  = 1 + 4 + 16 = 21

 6² +  3² +  1² = 36 + 9 + 1 = 46 =? ( The value of question mark) 

Option (3)46 is correct option

Problem # 8

 This reasoning problem also consists of three digits . In this problem we have to transform 124 to 69 using any rule in the same way we have to change 521 to a number out four given options. To solve this problem means to find the value of 2nd number in this figure , calculate  the sum of 1st digit , squares of middle digit and cube of 3rd digit .

Formula :- (1st digit)  +  (2nd digit)² + (3rd digit)³ 

1 +  2² +  4³  = 1 + 4 + 64 = 69

5 +   2² +  1³ = 5 + 4 + 1 = 10= ? ( The value of question mark) 

Option (4)10 is correct option

Also Reads these articles

Start

Latest-Questions-of-missing-numbers 

Maths logical reasoning Q & A

Reasoning for competive exams

Box and circle reasoning

 Reasoning for bank exams

Ten Tricky logical reasoning

Missing  number series questions

Reasoning questions with answers

Circle Reasoning

 Box Problems

15 Questions Circle Problems 

 SSC CGL Reasoning

End

Problem # 9

 In this reasoning problem 1st number (424) is associated to 96 with the help of any rule , in the same rule we have to associate 521 to a number out of four given option. These three digits can be utilised with the help a formula given below.

Formula :- (Two Right most digits  ×  Left most digit}

In this problem both the right most digits can be taken as single unit to multiply with the 1st number. 

4 × 24 = 96

5  × 21 = 105 = ? ( The value of question mark) 

Option (1)105 is correct option

Problem # 10

 In this reasoning problem 1st number (538) is associated to 725 with the help of any rule , in the same rule we have to associate 813 to a number out of four given option. These three digits can be utilised with the help a formula given below.

Formula :- (1st digit)  +  (2nd digit) + (3rd digit)

5  + 3 + 8 = 16
7  + 2 + 5 = 14 Sum of digits is 2 lees than above sum
8  + 1 + 3 = 12 
 We have to choose that option whose sum of digits must be 2 less than above sum. Therefore 
7  + 1 + 2 = 10 
Since all the three remaining option have total other than 10. 
8 + 1 + 4 = 13
2 + 1 + 9 = 12
3 + 2 + 8 = 13 = ? ( The value of question mark) 
Option (2)712 is correct option

Conclusion

Comment your valuable suggestion regarding the post most important Reasoning questions with answers which includes reasoning for competitive exams, circle problems, box problems, circle problems and triangles problems  for competitive exams like SSC CGL ,SSC CHSL ,CPO ,Bank exams and RRB NTPC etc which were explained in this post.

Read More

Maths logical reasoning questions with Answers

Most important Reasoning questions with answers which includes circle problems, box problems, circle problems, triangles problems  for competitive exams like SSC CGL ,SSC CHSL ,CPO ,Bank exams and RRB NTPC etc have been explained in this post. 

Ten Important Reasoning questions with answers for competitive exams

Problem #1 

This reasoning problem is a box problem of 4 rows and 3 columns, where the the value of question mark in last row we have to find. 

Formula :- 

Sum of the squares of 1st two columns = Squares of 3rd  column

1st Row

3² + 4² = 5²

➡ 9 + 16 = 25

➡ 25 = 25

L H S = R H S

2nd Row

6² + 8² = 10²

➡ 36 + 64 = 100

➡ 100 = 100

L H S = R H S

3rd Row

7² + 24² = 25²

➡ 49 + 576 = 625

➡ 625 = 625

L H S = R H S

4th Row

12² + ?² = 13²

➡ 144 + ?²  = 169

➡ ?²  = 169 -144

➡ ?²  = 25 , Taking square root on both sides

? = 5

Option (C)5 is correct option

Problem #2

Formula :- 

Five less than the product of sum of two corner's numbers in each rectangle. 

1st Rectangle

{(11 + 4) × ( 9 + 5 ) } - 5  = (15 × 14) - 5 = 210 - 5 = 205

2nd Rectangle

{(21 + 1) × ( 3 + 2 ) } - 5 = (22 × 5) - 5 = 110 - 5 = 105

3rd Rectangle

{(62 + 5) × ( 1 + 1 ) } - 5  = ( 67 × 2) - 5 = 134 - 5 = 129 = ? 

Option (2)129 is correct option

Problem #3

This reasoning problem consists of three triangles and every triangle have four numbers ,three around the triangle and one in the centre of the triangle. 

Formula :-  

Product of all the outer numbers  = Middle numbers

1st Figure

5 × 6 × 4 = 120 ⇒1 + 2 + 0 = 3 ( Middle number in 1st triangle ) 

2nd Figure

6 × 7 × 5 = 210  ⇒2 + 1 + 0 = 3 ( Middle number in 2nd triangle ) 

3rd Figure

4 × 8 × 10 = 320 ⇒3 + 2 + 0 = 5 ( Middle number in 3rd triangle ) 

Option (2)5 is correct option.

Problem #4

This reasoning problem consists of three squares and every squares have four small squares , And each small square contains a number in it. If we add all the numbers in each small square then we shall have total of 35 in each small square. 

Formula :- Sum of all the numbers in a square = 35

1st Square

5  + 12 + 8 + 10 = 35  

2nd Square

8 + 16 + 6 + 5 = 35

3rd  Square

4 + 10 + ? + 15 = 35

29 + ? = 35

? = 35 - 6

? = 6

Option (4)6 is correct option

Problem #5

1st Quadrant:-

HCF ( Highest common Factor ) of 4 and 8 is 4

2nd Quadrant:-

HCF ( Highest common Factor ) of 3 and 5 is 1

3rd Quadrant:-

HCF ( Highest common Factor ) of  6 and 12 is 6

4th Quadrant:-

HCF ( Highest common Factor ) of 10 and 25 is 5

Option (2)5 is correct option

Problem #6

All the numbers which are around the circle in any figure do not have any role to make the number in centre of the circle. But all the digits of the central number in 1st figure are reversed, So in same way all the digits of the central number in 2nd figure will be reversed to get the central/middle number in the 3rd figure. Hence 6543 will be the value of question mark. 

Option (4)6543 is correct option .

Problem #7

Sum of  all the numbers in any particular column are same for all column like

Formula :- 

All Numbers in any columns have equal total. 

[10 + 7](1st Column) = 17 = [9 + ? + 6](2nd Column)  = [ 15 +2] = 17( 3rd Column) . Now calculating the value of ? from any two column. 

→ ? = 2

Option (1)2 is correct option

 Also Reads these articles

Maths logical reasoning

Reasoning for competive exams
Box and circle reasoning
 Reasoning for bank exams
Ten Tricky logical reasoning
Missing  number series questions
Reasoning questions with answers
Circle Reasoning
 Box Problems
15 Questions Circle Problems 
 SSC CGL Reasoning

 Problem #8

Sum of squares of three numbers in upper line is equal to the number in lower line in each figure.

Formula :-  

Sum of the squares of numbers in 1st row  = Number in 2nd row 

1st Figure :-

2² + 5² + 6² = 4 + 25 + 36 = 65

2nd Figure :-

6² + 8² + 9² = 36 + 64 + 81 = 81

3rd Figure :-

2² + 8² + 7² = 4 + 64 + 49 = 117

Option (2)117 is correct option

Problem #9

In these types of reasoning questions either we can check the sum or product of all the numbers in any rows or column. If the sum or product of all the rows or columns is found to be same except the row or column in which question mark lies , then we can choose the value of question mark out of four given options to get the answer.

Sum of all the numbers in every row is 20.

2 + 3 + 7 + 8 = 20 ( Last Row ) 

4 + 7 + 9 = 20  ( 2nd Last Row ) 

11 + 9 = 20  ( 2nd Row ) 

 Hence the value of  "?"  must be 20

Option (4)20 is correct option. 

Problem #10

This Reasoning question have three triangles in it. And every triangle consists of four numbers around it , The middle number in each triangle can be written using the formula given below 

Formula :- b² - ( 1st side² - 2nd side²) = Middle number

1st Triangle :-

7² - ( 4² + 3²) = 49 - (16 + 9 ) = 49 -25 =  24  42

2nd Triangle :-

8² - ( 6² + 3²) = 64 - (36 + 9 ) = 64 - 45 =  19  91

3rd Triangle :-

9² - ( 5² + 3²) = 81 - (25 + 9 ) = 81 - 34 =  47 74

Option (3)74 is correct option. 

Conclusion

Comment your valuable suggestions regarding the post most important Reasoning questions with answers which includes reasoning for competitive exams, circle problems, box problems, circle problems and triangles problems  for competitive exams like SSC CGL ,SSC CHSL ,CPO ,Bank exams and RRB NTPC etc which were explained in this post.

Promoted content

 

Read More