Ten Missing term in box reasoning questions with answers

Ten Missing term in box reasoning , Reasoning questions with answer, box reasoning with solutions have been discussed in this post . These types of problems are very helpful for cracking competitive exams like ssc cgl, ssc chsl , RRB NTPC, Group D and various Bank exams and many other similar exams.

Missing term in box reasoning questions with answers

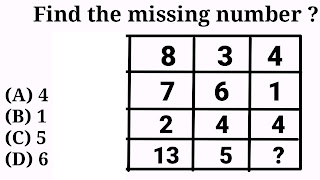
Problem # 1

Product of both the digits in 1st column is equal to sum of both the digits in 2nd column in every box. 

1st Box 

5 × 2 = 5 + 5 = 10

3 × 2 = 5 + 1 = 6

4 × 3 = 8 + 4 =12

2nd Box

5 × 2 = 6 + 4 =10

3 × 3 = 7 + 2 = 9 

4 × 4 = 7 + 9 =16 (choose one of the option from given four whose digits's product should be 16)

3rd Box

4 × 4 = 8 + 8 = 16

2 × 6 = 6 + 6 = 12 (choose one of the option from given four whose digits's sum should be 12)

3 × 5 = 6 + 9 =15

Hence correct option is (4)44, 66

Problem # 2

Sum of squares of both the digits in 1st column is equal to  the Number in 2nd column in every box. 

1st Box 

2² + 1² = 4 + 1 = 5

5² + 4² = 25 + 16 = 41

1² + 1² = 1 + 1 = 2

2nd Box

4² + 6² = 16 + 36 = 52

6² + 6² = 36 + 36 = 72

8² +  0² = 64 + 0 = 64

3rd Box

2² + 3² = 4 + 9 = 13

2² + 2² = 4 + 4 = 8

1² + 5² = 1 + 25 = 26

Hence correct option is (1)72, 13

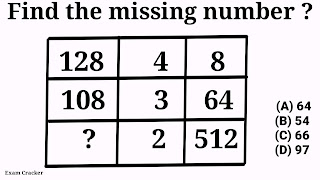
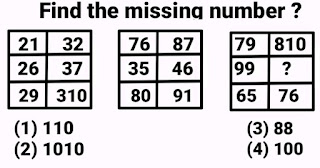
Problem # 3

Formula :-

Add one to both the digits in 1st column separately in every box to get the number in 2nd column in every box. 

1st Box 

2 + 1 = 3(Digit in ten's place) , 1 + 1 = 2(Digit in unit's place) 

Hence Number in 2nd column will be 32.

2 + 1 = 3(Digit in ten's place) , 2 + 1 = 7(Digit in unit's place) 

Hence Number in 2nd column will be 37.

2 + 1 = 3(Digit in ten's place) , 9 + 1 = 10(Digit in unit's place) 

Hence Number in 2nd column will be 310

2nd Box

7 + 1 = 8(Digit in ten's place) , 6 + 1 = 7(Digit in unit's place) 

Hence Number in 2nd column will be 87.

3 + 1 = 4(Digit in ten's place) , 5 + 1 = 6(Digit in unit's place) 

Hence Number in 2nd column will be 46.

8 + 1 = 9(Digit in ten's place) , 0 + 1 = 1(Digit in unit's place) 

Hence Number in 2nd column will be 91.

3rd Box

7 + 1 = 8(Digit in ten's place) , 9 + 1 = 10(Digit in unit's place). 

Hence Number in 2nd column will be 810.

9 + 1 = 10(Digit in ten's place) , 9 + 1 = 10(Digit in unit's place). 

Hence Number in 2nd column will be 1010.

6 + 1 = 7(Digit in ten's place) , 5 + 1 = 6(Digit in unit's place) 

Hence Number in 2nd column will be 76.

Hence correct option is (2)1010.

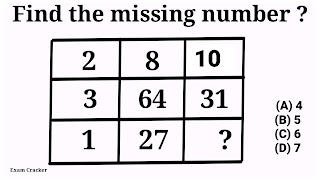
Problem # 4

 

Formula :-

Difference between the numbers in 1st and 2nd column is same in every row in all the three Boxes.

1st Box

54 - 50 = 4 (1st row) 

19 - 15 = 4 (2nd row)

43 - 39 = 4 (3rd row).

3rd Box

44 - 40 = 4 (1st row) 

70 - 66 = 4 (2nd row)

35 - 31 = 4 (3rd row)

2nd Box

34 - 30 = 4 (1st row) 

33 - 29 = 4 (2nd row)

? - 83 = 4 (3rd row).

? = 4 + 83

? = 87(The value of question mark). 

Hence correct option is (3)87.

Problem # 5

Formula :-

Sum of all the numbers in 1st column is 50 and Sum of all the numbers in 2nd column is 60 in all the three boxes. 

1st Box

14 + 13 + 23 = 50

18 + 15 + 27 = 60

3rd Box

22 + 18 + 10 = 50

20 + 16 + 24 = 60

2nd Box

16 + 13 + ? = 50

24 + 15 + 21 = 60

Hence ? = 50 - (16 +13) 

? = 50 - 29

? = 21

Hence correct option is (4)21.

Problem # 6

Formula :-

Sum of all the numbers in every  row is 100. 

1st Row

14 + 12 + 16 + 14 + 24 +20 = 100

2nd Row

19 + 15 + 13 + 18 + 19 +16 = 100

3rd Row

33 + 27 + 29 + ? + 5 +4 = 100

89 + ? + 9 = 100

?  + 98 = 100

? = 100 - 98

? = 2(The value of question mark) 

Hence correct option is (1)2.

Problem # 7

Formula :-

Product of both the digits in 1st column is equal to sum of both the digits in 2nd column in every box. 

1st Box 

5 × 2 = 5 + 5 = 10

3 × 2 = 5 + 1 = 6

4 × 3 = 8 + 4 = 12

2nd Box

5 × 2 = 6 + 4 = 10

3 × 3 = 5 + 1 = 6

4 × 3 = 8 + 4 = 12

3rd Box

5 × 2 = 5 + 5 = 6

3 × 2 = 5 + 1 = 6

4 × 3 = 8 + 4 =12

Hence correct option is (3)16.

Problem # 8

Formula :- 

The product of 1st and 3rd numbers in each column of every box is equal to the Number in middle number in opposite box.

1st Box

5 × 7 = 35 Number in middle row opposite to 2nd column (1st box 2nd column ) 

3 × 5 = 15 Number in middle row opposite to 1st column (1st box 1st column). 

2nd Box

4 × 2 = 8 Number in middle row opposite to 2nd column (2nd box 2nd column ). 

5 × 9 = 45 Number in middle row opposite to 2nd column ( 2nd box 1st column ). 

3rd Box

7 × 6 = 42 Number in middle row opposite to 2nd column (3rd box 2nd column ). 

8× 9 = 72 Number in middle row opposite to 2nd column ( 3rd box 1st column). 

Hence correct option is (3)42.

Problem # 9

  

Formula :- 

Number in middle row opposite to 2nd column

1st Box

5 + 7 = 12 Number in middle row opposite to 2nd column (1st box 2nd column ) 

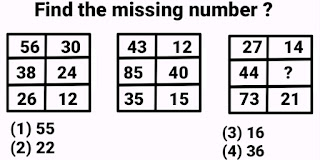
3 + 5 = 8 Number in middle row opposite to 1st column (1st box 1st column). 

2nd Box

4 + 2 = 6 Number in middle row opposite to 2nd column (2nd box 2nd column). 

5 + 9 = 14 Number in middle row opposite to 1st column (2nd box 1st column). 

3rd Box

7 + 6 = 13 Number in middle row opposite to 2nd column (3rd box 2nd column) . 

8 + 9 = 17 Number in middle row opposite to 1st column (3rd box 1st column). 

Hence correct option is (2)17.

Problem # 10

Formula :- 

Product of both the digits in 1st column is equal to product of both the digits in 2nd column in  every row and in every box . 

1st Box

5 × 4 = 4 × 5  = 20(1st row 1st box). 

1 × 2 = 2 × 1  = 2(2nd row 1st box). 

4 × 3 = 2 × 6  = 12(3rd row 1st box). 

2nd Box

3 × 4 = 6 × 2 = 12(1st row 2nd box). 

3 × 3 = 1 × 9 = 9(2nd row 2nd box). 

4 × 6 =  8 × 3 = 24(3rd row 2nd box). 

3rd Box

4 × 4 = 8 × 2 = 16(1st row 3rd box). 

6 × 4 = ? × ? = 24(2nd row 3rd box). 

So out of four option only the product of both the digits in 4th option is equal to 24.

3 × 5 = 5 × 3 = 15(3rd row 3rd box). 

Hence correct option is (4)38

Ten Missing term in box reasoning questions with answers box with solutions discussed in this post . These types of problems are very helpful for cracking competitive exams like ssc cgl, ssc chsl and various Bank exams and many other similar exams. please feel free to comment your opinions regarding this post.

Also Reads these articles

 Latest-Questions-of-missing-numbers 

Maths logical reasoning Q & A

Reasoning for competive exams
Box and circle reasoning
 Reasoning for bank exams
Ten Tricky logical reasoning
Missing  number series questions
Reasoning questions with answers
Circle Reasoning
 Box Problems
15 Questions Circle Problems 
 SSC CGL Reasoning

Read More

Different Types of matrix with examples

Types of matrix with definition , Different types of Matrix discussed in this post

Read More

Missing number in box Reasoning, Missing number in box puzzle

Ten most important missing number in box Reasoning, Missing number in box puzzle will be discussed with the help of ten most important examples. Some of these examples are of  3 × 3  order and other are of  4 × 4 orders.

Reasoning of missing number in box problems

 

Problem # 1

Exam Cracker

This box problem consist of four rows and four columns . And we have to find the value of question mark after studying the pattern of all the numbers in this box. This question mark is in 4th row of 4th column. 

     To find the value of question mark. we  shall divide this box into two parts vertically then we can have the formula for these numbers written in this box . Because after careful observation we can see that the product of both the numbers in left half in any particular row is equal to sum of both the numbers in right half in that particular row. 

Column wise :-

Formula:-  (1st number × 2nd number ) = (3rd number × 4th number) 

15 × 10 = 6 × 25 = 150 (Equal Product in both the half of 1st row)

4  × 16  =  8 × 8 = 64 (Equal Product in both the half of 2nd row)

6  ×  6 = 12 × 3 =  36 (Equal Product in both the half of 3rd row)

Similarly 

⇒  7 × 9 = 3 ×  ? = 63 (Equal Product in both the half of  4th row)

⇒  ? = 63

⇒  ? = 21

Option (B)21 is correct option.

 

Problem # 2

Exam Cracker

This reasoning problem consists of three figures and every figure have four numbers associated to it . Two numbers are on the upper line of each box and two number are at the bottom line of each box.  Look at last figure , it have ? in its 3rd figure . So the solution of this problem is to find the value of question mark using three numbers associated to it . 

          But the main problem is how to utilised  these three numbers to get the value of question mark?

          Now watch carefully the 1st two figures . Since these figures have some big values of numbers in one box . 

          Now we have to find or search the  formula for these four numbers in 1st two figures to utilised them in any possible way to get number in that box . 

         The same formula will be applicable to third figure to find out the value of question mark.

Formula:-  Product of all the numbers = 168 

1st Box 

Product of  all the numbers in 1st box is equal to 168

3 × 7 × 4 × 2 = 168 (1st figure) 

2nd Box 

Product of  all the numbers in 2nd box is equal to 168

8 × 3 × 7 × 1 = 168 (2nd figure) 

3rd Box 

Product of  all the numbers in 2nd box is equal to 168

1 × 6 × ? × 2 = 168 ( 3rd figure ) 

12 × ? = 168

? = 168 ÷ 12 

? =  14

Option (3)14 is correct option.

  

Problem # 3

Exam Cracker

This box problem consist of four rows and three columns . And we have to find the value of question mark after studying the pattern of all the numbers in this box. This question mark is in 4th row of 3rd column. 

     To find the value of question mark. we  shall divide this box into two parts horizontally then we can have the formula for these numbers written in this box . Because after careful observation we can see that the product of both the numbers in upper half in any particular column is equal to sum of both the numbers in lower half in that particular column. 

 Formula:-  (1st number + 2nd number )  =  (3rd number + 4th number) 

1st column :- 

8 + 7 = 2 + 13 = 15 (Equal sum in both the half of 1st column)

2nd column :- 

3 + 6 = 4 + 5 =  9  (Equal sum in both the half of 2nd column)

3rd column :- 

4 + 1 = 4 + ? = 5  (Equal sum in both the half of 3rd column)

⇒ 4 + ? = 5

⇒ ? = 5 - 4

⇒ ? = 1

Option (B)1 is correct option.

 

 Problem # 4

Exam Cracker

This box problem consist of three rows and three columns. And we have to find the value of question mark in 3rd row and 3rd column after studying the pattern of all the numbers in this box. 

             Third number of every row is equal to the sum of square of 1st number and square root of 2nd number.

Formula:-  Square of 1st number +  Square root of 2nd number

(4)²    +  √16 = 16 + 4 = 20  (Number in 1st row 3rd column ) 

(3)²    +  √36 = 9 + 6 = 15  (Number in 2nd row 3rd column ) 

(1)²    +  √25 = 1 + 5 = 6  (Number in 3rd row 3rd column ) 

Option (B)6 is correct option.

 
Problem # 5

This box problem consist of three rows and three columns. And we have to find the value of question mark after studying the pattern of all the numbers in this box.

      1st number of every row is equal to the product of square of 3rd number and square root of 2nd number.

Formula:-  Square of 3rd number  ×  Square root of  2nd number

(3)²    ×  √49 = 9 × 7 = 63 (Number in 1st row 1st column ) 

(4)²    × √25 = 16 × 5 = 80 (Number in 2nd row 1st column ) 

(?)²    ×  √64 = 200 (Number in 3rd row 1st column ) 

(?)²    ×  8 = 200

(?)²   = 200 ÷ 8

(?)²   = 25

?   = 5

Option (D)5 is correct option.

Problem # 6

Exam Cracker

This box problem consist of three rows and three columns. And we have to find the value of question mark after studying the pattern of all the numbers in this box.

    Since question mark is in the 3rd column of 3rd row, so we can use any two numbers in any two particular rows  to find the value of 3rd  number in that particular row.

Formula:-  Square of 1st number  +  (2nd number ÷ 2 ) = 3rd number 

(6)²   +  ( 8 ÷ 2 ) = 36 + 4 =  40 ( Number in 1st row 3rd column ) 

(4)²   +  (  6 ÷ 2 ) = 16 + 3 =  19 ( Number in 2nd row 3rd column ) 

(7)²   +  (  2 ÷ 2 ) = 49 + 1 = 50 ( Number in 3rd row 3rd column ) 

Option (D)50 is correct option.

Problem # 7

Exam Cracker

This box problem consist of three rows and three columns. And we have to find the value of question mark after studying the pattern of all the numbers in this box.

    Since question mark is in the 1st column of 3rd row, so we can use any two number in any two particular columns to find the value of 3rd  number in that particular column.

Formula:-  cube of 2nd number  ×  ( cube root of 3rd number ) = 1st number 

(4)³  ×  ∛8 = 64 × 2 = 128 ( Number in 1st row 1st column ) 

(3)³   ×  ∛64 = 27 × 4 = 108 ( Number in 2nd row 1st column ) 

(2)³   ×  ∛512 = 8 × 8 = 64 ( Number in 3rd row 1st column ) 

Option (A)64 is correct option.

Problem # 8

Exam Cracker

This box problem also consist of three rows and three columns. And we have to find the value of question mark after analysing the pattern of all the numbers in this box.

    Since question mark is in the 3rd column of 3rd row, so we can use any two number in any two particular rows to find the value of 3rd  number in that particular row.

Row wise

Formula:-  2 × (1st number - 2nd number ) = 3rd number 

( 14  - 10 ) × 2 = 4 × 2 = 8 ( Number in 3rd row 1st column) 

( 16  - 15 ) × 2 = 1 × 2 = 2 ( Number in 3rd row 1st column) 

( 19  - 13 ) × 2 = 6 × 2 =  ? = 12 ( Number in 3rd row 1st column) 

Option (D)12 is correct option.

Problem # 9

Exam Cracker

This box problem consist of three rows and three columns. And we have to find the value of question mark after studying the pattern of all the numbers in this box.

    Since question mark is in the 1st column of 3rd row, so we can use any two number in any two particular columns to find the value of 3rd  number in that particular column.

Column Wise

Formula:-  cube of 1st number  +  ( cube root of 2nd number ) = 3rd number 

(2)³   +  ∛8  = 8 +  2 = 10

(3)³   +  ∛64 = 27 +  4 = 31

(1)³   +  ∛27 = 1 + 3 =  4

Option (A)4 is correct option.

Problem # 10

 

Exam Cracker

This box problem also consist of three rows and three columns. And we have to find the value of question mark after analysing the pattern of all the numbers in this box.

    Since question mark is in the 2nd column of 3rd row, so we can use any two number in any two particular columns to find the value of 3rd  number in that particular column.

Row wise

Formula:-   (3rd number - 1st number ) = Middle number 

( 3/2 ) - ( 1 ) = 1/2  (Middle number in 1st row ) 

( 8/3 ) - ( 2 ) = 2/3  (Middle number in 2nd row )

( 19/5 ) - ( 3 ) = 4/5  (Middle number in 3rd row )

Option (D)4/5 is correct option.

Ten Most Important Missing number in box Reasoning, Missing number in box puzzle with solutions have been discussed in this post . These types of problems are very helpful for cracking competitive exams like ssc cgl, ssc chsl and various Bank exams and many other similar exams. please feel free to comment your opinions.

Also Reads these articles

 Latest-Questions-of-missing-numbers 

Maths logical reasoning Q & A

Reasoning for competive exams
Box and circle reasoning
 Reasoning for bank exams
Ten Tricky logical reasoning
Missing  number series questions
Reasoning questions with answers
Circle Reasoning
 Box Problems
15 Questions Circle Problems 
 SSC CGL Reasoning

Read More

Simplest and shortest Matrix method to solve linear equations of 3 variables

Matrix method to solve linear equations of 3 variables

In this post we are going to understand the concept of solving linear equations of three variables with the help of matrix method .

Matrix method to solve linear equations of three variables with the help of example. 

Set of given equations are 

x - y + z = 2    

2x - y = 0  

 2y - 2 z = 1  

Rearranging these equations in symmetrical form. It means if any one of the variable in any equation is missing then write that missing variable/s with zero coefficient. As we see in this case the coefficient of z in 2nd equation and the coefficient of x in 3rd equation are missing. So we have to write coefficient of x in 2nd equation and coefficient of x in 3rd equation as zero.

x - y + z = 2 ----------------------> (1)

2x - y + 0z = 0 ------------------> (2)

0.x + 2y - 2z = 1 ----------------> (3)

The system of these equations can be transformed into Matrix form .

AX = B , ⇒ X = A^-1B  -------> (*)

Where A is matrix written from the coefficients of x, y and z when these equations are in symmetric form and B is the matrix written from constants from right hand sides in column form and X is matrix of all the variables in column form.

In order to find the solution of set of these equations , first we have to find the inverse of matrix A if it exist then we can find the solution otherwise Matrix method fails to find the solution of the set of linear equations . 

Evaluation of Determinant 

                                                  

|A| = 4 (18 - 4) -0(9 - 3) +6(12 - 18)

       = 4(14) + 0 + 6(-6) 

       = 56 - 36

        = 20

Since the determinant value of this matrix is not equal to zero ,Therefore its inverse can be calculated.

And formula for finding the inverse of matrix A is

Where Adjoint A is the transpose of co factor matrix. And in order to find the co factor matrix of any matrix, we have to find co factors of all the elements present in this matrix. 

How to calculate  co factors of all the elements of the matrix A

Let us calculate these cofactors.  

Now these co factors can be written in matrix form known as co factor Matrix. 

Co factors of 1st row are  (18 - 4) , -(9 - 3), (12 - 18) 

i. e. Co factors of 1st row are 14, -6 , -6

Co factors of 2nd row are -(0 -24), (12 - 18) , -(16 - 0) 

I. e. Co factors of 2nd row are  24 , -6 , -16

Co factors of 3rd row are  (0 - 36), -(4 - 18), (24 - 0) 

i.e. Co factors of 3rd row are  -36 , 14 , 24

Co factor Matrix

Writing co factors of 1st row in 1st row of this matrix , co factors of 2nd row in 2nd row of this matrix . Similarly co factors of 3rd row in 3rd row of this matrix . 

 

Adjoint  Matrix

To find the Ad joint of this matrix we have to take it's transpose, Because transpose of any matrix is called Ad joint of the matrix. So writing all the elements which are in 1st row in 1st column, and  all the elements which are in 2nd row in 2nd column and  all the elements which are in 3rd row in 3rd column. 

Inverse  Matrix

Now we can find inverse of the matrix A by putting the value of inverse of A in equation  (4), Now  putting the values  Matrix B and    A^-1  in (4) After simplification and using the properties of equality of two matrices  ( Two matrices of same order are equal iff their respective elements are equal to each other ) 

Now we shall use the property of equality of two matrices ,which says that if two matrices are equal to each other then their respective elements must be  equal to each other.

⇒ x = 10

    y = 10

    z = 10

So this was the Matrix method of solving linear equations of three variables using inverse of matrix. Your valuables comments will be appreciated for betterment of this blog.

Read More