Number Analogy Reasoning questions with answers for competitive exams
Ten Most Important Number Analogy Reasoning questions with answers for competitive exams have been discussed in this post . These types of problems are very helpful for cracking competitive exams like ssc cgl, ssc chsl and various Bank exams and many other similar exams. please feel free to comment your opinions about this post .
Number Analogy Reasoning questions with answers for competitive exams
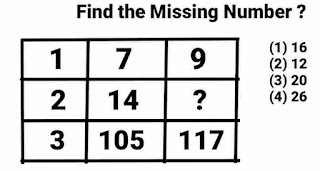
Problem # 1
Formula:-
1st Row
2nd Row
3rd Row
4th Row
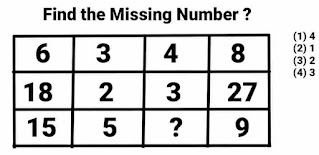
Problem # 2
Formula:-
Problem # 3
Problem # 4
In this problem of reasoning we have to combine both the given numbers in left hand side in such a way that after applying any mathematical operation/operations we could get the number on the right hand side of all the three problems given above. Because in these types of reasoning problems we can change given mathematical sign according to our requirements.
Formula:-
1st Problem
2nd Problem
3rd Problem
Problem # 5
In this problem of reasoning we have to combine both the given numbers in the left hand side in such a way that after applying any mathematical operation/operations we could get the number on the right hand side of all the three problems given above.Formula:-
1st Problem
2nd Problem
3rd Problem
Problem # 6
Formula:-
1st Pair
2nd Pair
3rd Pair
3rd Pair
Problem # 7
1st Method
2nd Method
All the three numbers given in this problem are prime numbers so fourth will also be prime number. Therefore only one prime number out of given four options is 5.
Formula
Problem # 9
Formula :-
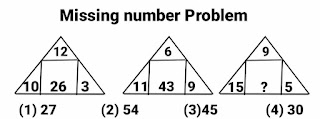
1st Triangle
12 × 3 = 26 + 10 = 36
2nd Triangle
6 × 9 = 43 + 11 = 54
3rd Triangle
9 × 5 = ? + 15 = 30
? + 15 = 30
? = 30 - 15
? = 25
Therefore correct option is (4) 25
2nd Method
Formula :-
1st Triangle
{( 12 × 3 ) - 10 } = ( 36 - 10) = 26
2nd Triangle
{( 6 × 9 ) - 11 } = ( 54 - 11) = 43
3rd Triangle
{( 9 × 5 ) - 15 } = ( 45 - 15) = 30
Therefore correct option is (4) 30




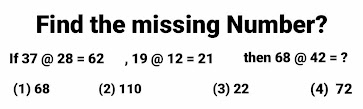

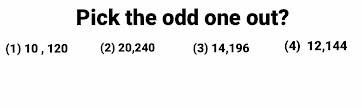







.jpg)








_11zon.jpg)
_11zon.jpg)